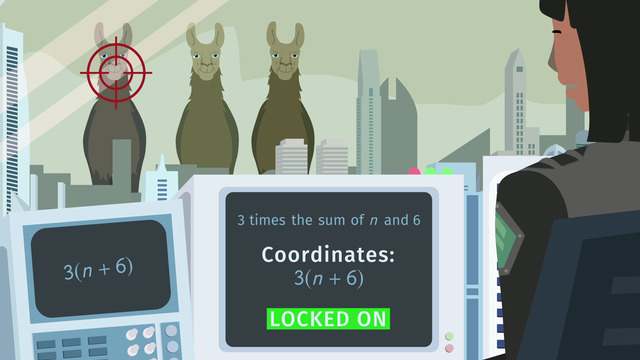
How to Write Expressions
- How to Write Expressions
- How to Write Expressions – Definition
- How to Write Expressions – Step-by-Step Process
- Step-by-Step Process of Writing an Algebraic Expression


Basics on the topic How to Write Expressions
How to Write Expressions
Writing mathematical expressions is a fundamental skill that helps translate real-world situations into manageable mathematical problems. It involves identifying key quantities and their relationships and then using symbols and numbers to express these relationships succinctly. This guide will explore the basics of constructing mathematical expressions, equipping you with the tools to solve problems efficiently and accurately.
How to Write Expressions – Definition
An algebraic expression is a mathematical phrase containing numbers, variables, and operation symbols. In business planning, algebraic expressions can model financial scenarios, calculate costs, and predict growth.
Here's a table summarizing common keywords associated with each of the basic mathematical operations, which can help in identifying what operation to use when writing mathematical expressions:
| Operation | Keywords |
|---|---|
| Addition | add, plus, increase, total, sum, together, more than |
| Subtraction | subtract, minus, less, decrease, fewer, less than |
| Multiplication | multiply, times, product, doubled, tripled |
| Division | divide, divided by, quotient, per, ratio |
How to Write Expressions – Step-by-Step Process
Here's a concise step-by-step guide on how to write an algebraic expression, followed by two simple examples to demonstrate the process:
Step-by-Step Process of Writing an Algebraic Expression
| Step | Description |
|---|---|
| Step 1: Identify the Variables | Determine what the unknowns are in the problem and assign a variable (like $ x $, $ y $, etc.) to each. |
| Step 2: Determine the Operation | Based on the keywords or problem context, decide which mathematical operations (addition, subtraction, multiplication, division) are required. |
| Step 3: Formulate the Expression | Combine the variables and numbers using the operations identified to create an algebraic expression that represents the problem. |
Let’s look at some examples.
Example 1:: Suppose you have 5 dimes and you receive $ x $ additional dimes from a friend.
- Identify the Variables: Let $ x $ represent the number of dimes received.
- Determine the Operation: The problem involves adding the dimes you received to the ones you already have.
- Formulate the Expression: The algebraic expression for this situation is $ 5 + x $.
Example 2:: You buy $ y $ pencils, and each pencil costs 3 dollars.
- Identify the Variables: Let $ y $ represent the number of pencils.
- Determine the Operation: Since each pencil costs a certain amount, you multiply the number of pencils by the cost per pencil.
- Formulate the Expression: The algebraic expression for this situation is $ 3y $.
How to Write Expressions – Real-World Application
Example: For Mateo's taco catering business, he has a simple pricing model. He sells each taco for $\$$2.50 and charges an additional service fee of $\$$200. An algebraic expression to represent Mateo's total charge for 't' tacos is:
$2.50t + 200$
With '$t$' representing the number of tacos sold, we can calculate Mateo's revenue by substituting the value of '$t$' into the expression.
Mateo serves three tacos per person and needs to account for kitchen accidents that usually cost him 10 tacos. To calculate the number of people Mateo can serve, we use the following expression:
$(t - 10) \div 3$
This expression takes the total number of tacos '$t$', subtracts the $10$ accident-prone tacos, and divides by $3$ since each person gets three tacos.
Mateo projects that his company's value will double each week. To model this exponential growth, we use the following expression:
$10,000 × 2^{w}$
Here, 'w' represents the number of weeks, and the entire expression predicts the company's value after 'w' weeks.
How to Write Expressions – Guided Practice
1. Hannah makes and sells handmade necklaces. She charges $\$$15 for each necklace and a fixed shipping fee of $\$$5 per order.
Algebraic Expression: The total charge for 'n' necklaces would be: $ 15n + 5 $
With '$n$' representing the number of necklaces sold, we can calculate Hannah's revenue by substituting the value of '$n$' into the expression.
2. Alex tutors students and charges $\$$20 per hour. Additionally, he charges a one-time material fee of $\$$10 per student.
Algebraic Expression: The total charge for 'h' hours of tutoring would be: $ 20h + 10 $
With '$h$' representing the number of tutoring hours, we can calculate Alex's total earnings by substituting the value of '$h$' into the expression.
How to Write Expressions – Practice
How to Write Expressions – Summary
Key Learnings from this Text:
- Algebraic expressions can simplify financial calculations in business planning.
- Translating business scenarios into algebraic expressions is essential for strategic planning and forecasting.
Engage further in exploration of the interplay between algebra and real-world applications like business planning, through our engaging content, interactive practice problems, and additional learning resources on our platform.
How to Write Expressions – Frequently Asked Questions
Transcript How to Write Expressions
Loco Mateo is crazy for tacos, so he decides to start a catering service which will feature his signature creation: Tacos el Fuego. Because he knows his tacos are so insanely delicious, he’s absolutely certain that his new business is going to be a success. So, he needs to write up a business plan to make sure he’s ready for all the HUGE orders he’s definitely going to get once he launches. To help him prepare for his inevitable financial success, Mateo is going to have to enlist the help of some basic algebraic expressions. Mateo’s plan is to cater to weddings, funerals, family reunions..anywhere there is a big group of people fiending for tacos. Since each group is going to be different, he needs to come up with an expression to represent how much he'll charge for each catering order, based on the number of tacos sold. Mateo decides to charge $2.50 for each Taco el Fuego plus, he’s going to charge a $200 service fee for his impeccable taco servicing skills. By focusing in on the keywords, we can turn Mateo's business plan into an algebraic expression. Since each taco costs $2.50, we should multiply 2.50 by 't', or the number of tacos sold, to get the total amount of money Mateo can earn by selling tacos. The variable 't' represents the number of tacos Mateo sells. When multiplying by a variable, you can either use a multiplication sign or just put the number right next to the variable. Finally, we know that the keyword "plus" indicates addition, so we add a plus 200 to complete the expression. Next, Mateo is going to need to figure out how many people he can feed with each batch of tacos he makes. Because he wants to keep his business exclusive, he decides to limit the number of tacos to three per person. But, Mateo is also not the most efficient chef. Every time he prepares a batch of tacos, he always burns or breaks some of them, so he ends up with 10 fewer than the total number of tacos in the batch. Let's use this information to write an expression. We knows that Mateo will end up with 10 fewer tacos than the total number of tacos he makes. We don't know how many the total will be, so we can use the variable 't' to stand for the total number of tacos. The words "fewer than" tell us that we are going to need to subtract 10 from the total, 't'. We also know that he will serve three tacos per person. The keyword "per" tells that we need to set up a division problem. So we take the total number of tacos remaining after the 10 misfires... Then divide that all by three. There! Now when we know the number of tacos, 't', we can use this expression to figure out how many people Mateo can feed! Now that he’s got a basic business model in place, Mateo just KNOWS that stocks in his company are going to soar! Right now, he figures that it’s worth about $10,000 but if everything goes according to plan, his company will double in value every week. How can we use this information to come up with an expression? Let's start with the initial value of the company, $10,000. Next, we have the keywords "double each week", which means we're going to do repeated multiplication by 2 every week. Let's draw up a quick table. At the end of week one, we know the company will be worth $10,000 x 2, or $20,000. At the end of week two, it will be worth $10,000 x 2 x 2, or $40,000. At the end of week 3, it will be worth $10,000 x 2 x 2 x 2, or $80,000. Do you notice a pattern here? Each of these expressions can be rewritten using exponents. $10,000 x 2 can be written as $10,000 x 2 to the first power. $10,000 x 2 x 2 can be written as $10,000 x 2 to the second power. Similarly, $10,000 x 2 x 2 x 2 can be written as $10 x 2 to the third power. Using this pattern, we can calculate how crazy rich Mateo’s going to be on week w, because we know that we'll have to multiply $10,000 by 2, "w" times! We can write this as the expression, $10,000 x 2 to the 'w' power! It looks like Loco Mateo has just finished up a batch of Tacos el Fuego in time to fill his first order! But where are all of his customers? I guess that’s why they call him Loco!
How to Write Expressions exercise
-
Explain how to identify the operator by keywords.
HintsKeep the keywords for the operations in mind:
- plus or more than or combine or in total ... for addition.
- minus or less than or fewer than or take away ... for subtraction.
If each student has $2$ books, and letting $s$ stand for the number of student there are in total, then the total number of books is given by $2s$, or $2\times s$.
Imagine you've got $12$ candies. You want to split those candies into packages of $3$ each.
Then you have to divide $12\div 3=4$ to see that you get $4$ packages with $3$ candies each.
SolutionSo let's help Mateo with his business plan:
First, he establishes the following:
- $2.50$ dollars for each taco
- a delivery fee of $200$ dollars
For each delivery, Mateo decides to charge a flat rate delivery fee of $200$ dollars. We know that plus stands for addition, so we have to add $200$ dollars to the term $2.50t$ to get
$2.50+200$.
Next, we take a look at the number of people he can feed with tacos:
- Each person gets 3 tacos.
- Mateo can only calculate with 10 fewer than the total number of tacos.
Fewer than indicates subtraction. So we have to subtract $10$ from $t$. Keep the order in mind. This gives us $t-10$.
So the remaining number of tacos is given by $t-10$.
Because each person gets 3 tacos we have to divide $t-10$ by $3$ to end up with the expression
$\frac{t-10}3$.
-
Decide the operation symbol.
HintsTake care: The number of persons can't be negative.
Keep the keywords for the operation symbols in mind:
- plus or combine ... for addition
- minus or fewer than ... for subtraction
- for each or times ... for multiplication
For example, the total of $a$ and $b$ can be written as
$a+b$.
SolutionTo write a word problem as an algebraic expression, you have to recognize keywords. In the following the keywords are highlighted.
Cheyenne has one sibling and Lou has two siblings more than Cheyenne. Thus we have to add:
$1+2$.
So, in other words, Lou has three siblings.
Cheyenne has ten apples fewer than Lou. Let the variable $l$ represents the number of Lou's apples. The keyword indicates subtraction. So the corresponding expression is
$l-10$.
Together Cheyenne and Lou would like to solve ten word problems. They want to split the problems evenly. To split evenly means division:
$10\div 2=5$.
So each of them has to solve five word problems.
Well done Cheyenne and Lou: now you can order $t$ tacos, at $3$ dollars each. Together they have to pay
$3\times t$ dollars.
-
Match the keyword with the operation symbol.
HintsTake any of the given keywords and think about which operation symbol you have to take, and test out what you think by making up a word problem to go along with it.
Keep in mind:
- Summand $+$ summand $=$ sum
- Minuend $-$ subtrahend $=$ difference
- Factor $\times$ factor $=$ product
- Dividend $\div$ divisor $=$ quotient
SolutionLet's start with the addition sign $+$:
The following keywords in a word problem indicate addition: combine, in total, sum, plus, more than ... Sure, there are a lot more keywords for addition as well.
Now we take a look at subtraction, the $-$ sign:
Keywords you usually find are difference, take away, less than, fewer than, minus ...
Multiplication can be indicated by the following keywords:
for each or times or product. For example, the double of can be written as $2\times ...$.
Last, the division keywords:
split, divide, as well as relation
-
Highlight important keywords from various real world statements.
HintsYou only have to highlight the keyword and do not have to decide the operation.
Be careful: there are also non keywords that you can highlight.
If any word is a keyword, you should be able to write down a corresponding algebraic expression.
SolutionFirst, we look at the keywords and then we indicate the corresponding operation and write down the algebraic expression.
Here only the keywords are highlighted. If any word isn't highlighted, then it isn't a keyword at all.
$~$
Shells
The total number of shells is wanted. So we have to add the found shells to get $23+16=39$.
$~$
Classrooms
Because $20$ students can fit in each classroom, we have to divide $320\div 20=16$. This is the number of classrooms the school needs.
The next example is more theoretical:
$t$ raised to the fourth power leads to $t^4$. Next it's increased: this indicates addition of the product of $2$ and $s$. So we have in total $t^4+2\times s$.
$~$
Books
Sasha has nine books. Chayenne has two times more books than Sasha.
- two times stands for multiplication
- more than stands for addition
$9+2\times 9=27$.
This is the number of books Chayenne owns.
-
Summarize your knowledge about variables.
HintsIf you don't know the number of students in your grade, you can represent the unknown number by a variable, such as $s$.
If you've decided one letter for an unknown value you have to use it consistently throughout the exercise.
Let's have a look at the algebraic expression, $x+4$.
- $x=2$ we get $2+4=6$
- $x=10$ we get $10+4=14$
- ...
SolutionIf you'd like to write an algebraic expression with an unknown value, you first assign a variable to this unknown value.
Often the variable $x$ or $y$ is used, but sure you can also use any letter. For example, $t$ for the number of tacos.
If you've decided on using one variable you don't have to change it during an exercise.
Let's have a look at the example of Matteo's taco company:
$2.50t+200$
is the expression for the money coming into Matteo's company. Now, Matteo is able to put different values in for $t$ to get:
- $2.50\times 100+200=250+200=450$ for $t=100$
- $2.50\times 400+200=600+200=800$ for $t=400$
- ...
-
Determine the corresponding algebraic expressions.
HintsThe total numbers of people is four - including Sasha.
The total number of tacos is $12$ and the total number of drinks is $10$.
The total bill is $61$ dollars.
SolutionIt's also quite a good strategy to practice word problems in the real world. So, Sasha and her friends do it. Mathematics in a taco bar:
The number of eaten tacos
Each of them has eaten three tacos. Together we get $4\times 3=12$ tacos eaten.
The number of drinks
Two had two and the other two had three drinks. So we have to multiply two times and to add the products last:
$2\times 2+2\times 3=4+6=10$, the total number of drinks.
They ask for the bill
$10$ tacos, $3$ dollars each, and $10$ drinks $2.50$ dollars each leads to
$12\times 3+10\times 2.50=36+25=61$ dollars. So they have to pay $61$ dollars in total.
They'd like to pay
They increase the total bill by $3$ dollars tip, $61+3$, and split this sum evenly to get
$\frac{61+3}4=\frac{64}4=16$.
So each of them has to pay $16$ dollars.