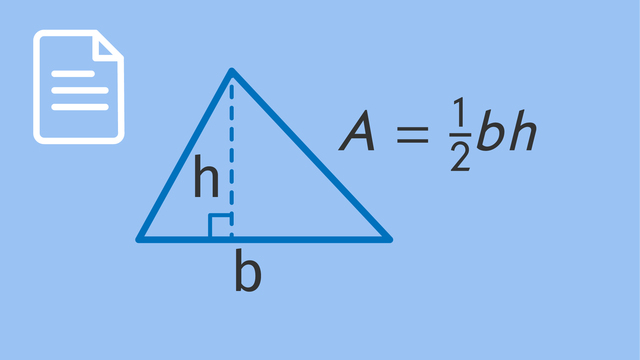Finding the Area of an Obtuse Triangle
Basics on the topic Finding the Area of an Obtuse Triangle
After this lesson, you will understand and apply the area formula for an obtuse triangle.
The lesson begins with a review of how to find the areas of right and acute triangles. It then relates the area of an obtuse triangle to half the area of a parallelogram. It concludes with an example of how to apply this area formula, then check your answer with decomposition.
Learn about the area of obtuse triangles by helping Captain Schneider generate electricity from sails!
This video includes key concepts, notation, and vocabulary such as: right triangles (with one right angle); acute triangles (with all angles less than 90 degrees); obtuse triangles (with one angle greater than 90 degrees; rectangles (with an area of base times height); parallelograms (also with an area of base times height); the area of a triangle (half of base times height).
Before watching this video, you should already be familiar with types of triangles, the area of rectangles and parallelograms, and the area of right and acute triangles.
After watching this video, you will be prepared to learn how to find the area of more complex figures through composition and decomposition.
Common Core Standard(s) in focus: 6.G.A.1 A video intended for math students in the 6th grade Recommended for students who are 11 - 12 years old
Transcript Finding the Area of an Obtuse Triangle
Captain Schneider has spent most of his life sailing the seven seas. He's had adventures...survived danger...and romance. But now that he's retiring, it's time to return to life among the landlubbers in his hometown of Polygon, Pennsylvania. Captain Schneider wants to use his sailing experience to contribute to the mayor's Revitalize the City project by building sails which will collect wind-energy and convert it to electricity! But if he wants his project to fly he'll need to find the area of obtuse triangles. All of Captain Schneider's wind-energy collectors are shaped like triangles--just like sails on a ship! To figure out how much material he needs to build them, we're going to have to find their areas. Triangles which have one right angle, are called right triangles. If we call the base of this triangle 'b' the height, 'h', will be the side that is perpendicular to the base. How can we use the base and height to find the area of this triangle? Remember, a right triangle is half of a special kind of parallelogram known as a rectangle. Like all parallelograms, a rectangle's area is found by multiplying its side lengths together. So we can find the area of a right triangle by multiplying, one-half the base times the height. Now let's look at a second kind of triangle. Triangles shaped like this are called "acute", because their angles are all less than 90 degrees...and isn't that cute? The height is still perpendicular to the base, and we find it INSIDE the triangle. So how do we use that to find the area of this shape? If we DECOMPOSE this triangle along the height, so that there are two RIGHT triangles, we can see that the area of the triangle on the left is half the area of a rectangle and the area of the triangle on the right is also half the area of a rectangle. So the area of an acute triangle is half of the area of the rectangle which circumscribes it. That makes the area formula for an acute triangle the same as the formula for a right triangle: Area equals one half the base times the height. But not all of Captain Schneider's wind-collectors are shaped like right and acute triangles. Some of them are triangles with one angle GREATER than 90 degrees. These triangles are called OBTUSE, and they have a lot in common with acute and right triangles. For starters, they all have a base, which we can call, 'b'. They also have a height which is perpendicular to the base. Where would you draw the height on this triangle? If we imagine extending the base we can measure the height, 'h', OUTSIDE the triangle's area. Now, how can we use this information to calculate the area for Captain Schneider's obtuse wind-collectors? Remember that right and acute triangles are half of the area of a specific kind of parallelogram: a rectangle. Can you visualize a PARALLELOGRAM we can form, using this obtuse triangle? If we rotate it around we can see that our obtuse triangle is half the area of a parallelogram! So, in order to find the area of this obtuse triangle, or ANY triangle, all we need to do is multiply one half the base times the height! Keep that in mind, as we apply this formula to one of Schneider's wind-energy sails. In order to find the area of this obtuse triangle, which number should we use for our base? Well, this side is on the bottom, so let's substitute 24 feet for our base, 'b', in the formula. Notice that we just need the length of the SIDE we are calling the base, NOT the additional length outside of the triangle. Now, which number should we use for our height? The height of any triangle is the perpendicular distance from the base to the top of the triangle which makes our height, 'h', 42 feet. That makes the area one-half of 24 times 42, or 504 square feet. While the Captain gets everything in ship-shape let's review. No matter if a triangle is right, acute, or obtuse in order to calculate the area, we just need to multiply one half the base times the height. To find the height, make sure that you are measuring a perpendicular line which extends from the base to the top of the triangle. And when substituting into our formula, remember that you don't necessarily need to use ALL measurements that you are given--just the base and the height. With Captain Schneider's new wind energy system in place, the mayor's Revitalize the City project is ready for take-off! Uh, is that how these things are supposed to work?
