Finding the Area of an Acute Triangle
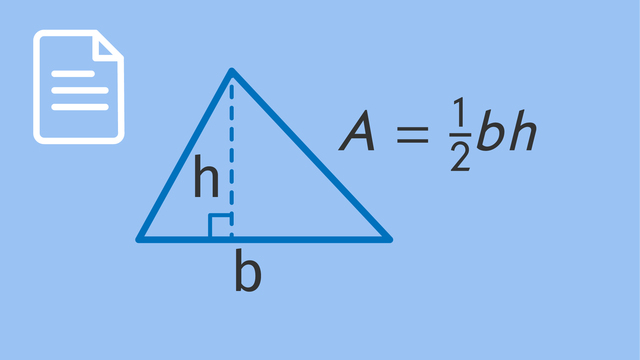

Basics on the topic Finding the Area of an Acute Triangle
Finding the Area of Acute Triangles
In this text, we're learning how to find the area of acute triangles. These are triangles where all the angles are smaller than 90 degrees. Knowing how to do this is useful, not just in math class but for real-life stuff too, like planning out a garden or figuring out the shape of a sail.
Plus, there's a cool thing about triangles: whether they are acute, obtuse, or right triangles, you can use the same simple formula to figure out their area. This makes understanding triangles a bit easier and shows how math can be pretty handy in the real world.
An acute triangle is defined by having all three interior angles less than 90 degrees.
Classifying triangles by angles is necessary when distinguishing between acute, obtuse, and right triangles.
Types of Acute Triangles
Acute triangles can be further classified based on their side lengths. This classification helps in understanding the properties and solving problems related to these triangles more effectively. Here is a brief overview:
| Type | Description | Characteristics |
|---|---|---|
| Equilateral Acute Triangle | All three sides are of equal length, and all three angles are equal, measuring 60 degrees each. | - All sides equal - All angles are 60° |
| Isosceles Acute Triangle | Two sides are of equal length, and the angles opposite these sides are also equal. Typically, these triangles have two acute angles and one angle that could be acute or obtuse, but in the context of an acute triangle, all angles are less than 90 degrees. | - Two sides equal - Two angles equal |
| Scalene Triangle | All three sides and all three angles are of different lengths and measures, respectively. | - No sides are equal - No angles are equal |
Area of a Triangle Formula
The area of an acute triangle is found using the formula: $A=\frac{1}{2}bh$, where $b$ is the base's length, and $h$ is the height, measured perpendicularly from the base.
Base and Height of Acute Triangles
In geometry, especially when working with triangles, two key terms we often encounter are the base and the height. Understanding these terms is crucial for solving various problems, including finding the area of triangles.
Base: The base of a triangle is any one of its sides that we use as a reference point for measurement. When calculating the area, you can choose any side as the base, but typically, it's the bottom side when the triangle is drawn on paper.
Height: The height (or altitude) of a triangle is a perpendicular line segment from the base to the opposite vertex. The height measures how tall the triangle is from its base to its highest point.
Finding the Area of an Acute Triangle – Step-by-Step Process
| Step | Description | Example |
|---|---|---|
| 1. Identify the Formula and Base/Height | Start by recognizing the formula for the area of a triangle, which is $A = \frac{1}{2}bh$. Then, identify which side of the triangle will serve as the base ($b$) and the perpendicular height ($h$) from that base. | For our example, let's use a triangle with a base ($b$) of 8 cm and a height ($h$) of 5 cm. |
| 2. Substitute the Values into the Formula | Plug the values of the base and height into the formula to prepare for calculation. | Substitute the values into the formula: $A = \frac{1}{2}(8 \text{ cm})(5 \text{ cm})$. |
| 3. Calculate and Add Units for the Final Answer | Perform the multiplication and division as indicated by the formula to find the area. Remember to include the correct units in your final answer, which will be in square units. | Calculate the area: $A = \frac{1}{2}(8 \text{ cm})(5 \text{ cm})$ $A=\frac{1}{2}(40 \text{ cm})$ $A=20 \text{ cm}^2$ The area of the triangle is $20 \text{ cm}^2$. |
Let’s look at another example.
Find the area of the triangle.
Finding the Area of an Acute Triangle – Practice
Use the steps to find the area of more acute triangles on your own!
Acute Triangle Area – Summary
Key Learnings from this Text:
- The formula $A=\frac{1}{2}bh$ is essential for calculating the area of an acute triangle.
- Base is defined as any side of the triangle that is considered the reference or bottom side for the calculation.
- Height is the perpendicular distance from the base to the opposite vertex, crucial for determining the triangle's area accurately.
- Understanding and applying this formula enhances geometric understanding and problem-solving skills.
- Regular practice with triangles of various sizes and configurations builds proficiency and confidence in these calculations.
After you have learned about finding the area of acute triangles, learn more about finding the area of an obtuse triangle.
Acute Triangle Area – Frequently Asked Questions
Transcript Finding the Area of an Acute Triangle
Flying above the city of Polygon, Pennsylvania Caroline the Consultant sees an opportunity to add some flair to the city's skyline. The tops of Polygon's triangular skyscrapers are just so plain. What a waste of primo advertising space! So Caroline the Consultant brings a design plan to the mayor of Polygon. Why not turn these ugly old rooftops into sky-high welcome mats, greeting visitors to the city! To design effective advertisements for each skyscraper, we'll have to measure the size of their rooftops which means finding the area of acute triangles. The tops of both skyscrapers are shaped like acute triangles. Acute triangles are triangles where ALL the angles are smaller than 90 degrees. Another kind of triangle--which has exactly one 90 degree angle--is called a RIGHT triangle. A right triangle looks a bit like a rectangle, if it was cut in half with a diagonal. So how would you calculate the area of a right triangle? Since right triangles are half of a rectangle, we can find their area by multiplying one half the length of the base times the height. Let's see if we can use this information to figure out the area of our acute rooftops. Look closely at the acute triangle on the left: can you see a way to break this up into RIGHT triangles? If we draw a line perpendicular to the base, we can create two separate right triangles. Notice that this line is the height of both right triangles and the acute triangle itself because it is perpendicular to the bases of each. Now to find the area of the acute triangle, all we have to do is add together the areas of these two right triangles. Let's start with the triangle on the left: what numbers should we use for our base and height? The length of the bottom side is 20, so we'll use that for our base. The line we drew perpendicular to that side has a length of 10, so we'll use that for our height. Multiplying, we see this triangle takes up 100 square meters. The triangle on the right has a base of 4 and a height of 10, giving it an area of 20 square meters. If we add the areas of both triangles together, the total area is 120 square meters. Before we move on, let's see if we can find a more general formula to make our work easier in the future. Notice that the base of the whole acute triangle is 24. If we substitute that and the height of 10 into our area formula, we get the same answer! That means that just like right triangles, the area of our acute triangle is ALSO half the area of a rectangle with the same base and height. To understand WHY this works, let's circumscribe a rectangle around our acute triangle. With our height line still drawn, we now have four right triangles. The two right triangles on the left make up two halves of a rectangle and the two triangles on the right form another rectangle. So our original triangle takes up exactly half the space of a rectangle with the same base and height which we can see here as two identical acute triangles. This is why the area of any acute triangle will always be one half the base times the height. Let's keep this in mind, as we calculate the area of the second rooftop. In order to use our area formula, we need to find the base and height of this acute triangle. So what number should we use for our base? This ENTIRE side is our base, so we're going to have to combine 18 and 22 to get the WHOLE side length of 40 meters. We can substitute that into our formula for the base, 'b'. Now which number should we use for the height? When looking for the height, always keep an eye out for the right angle symbol, which indicates that two lines are perpendicular. That makes our height, 'h', 24. Multiplying together we see this rooftop has an area of 480 square meters! While those welcome signs are being built, let's review. Just like with right triangles, to find the area of an acute triangle, we can use the formula, one-half base times height. This works, because every acute triangle is composed of two right triangles which are themselves each one half of a rectangle. Finally, when identifying the base and height of any triangle, make sure they are perpendicular to each other and be sure to use a WHOLE side length for your base. Wow, these new signs are going to be GREAT for Polygon's tourist industry! Just wait until word gets out that everyone is welcome here in Polygon...EVERYONE.
Finding the Area of an Acute Triangle exercise
-
Understand the characteristics of an acute triangle.
HintsThe sum of the three angles in a triangle is always $180^\circ$.
The measurements of these angles can determine the type of triangle:
- acute triangle (pictured below)
- right triangle
- obtuse triangle
Obtuse triangles have one angle greater than 90 degrees.
Right triangles have one angle equal to 90 degrees.
SolutionAn acute triangle is a triangle with all angles less than $90^\circ$.
-
Identify the elements in the formula for the area of an acute triangle.
HintsThe height of an acute triangle is the straight line drawn from one vertex (corner) to the opposite side, forming a right angle with that side. It measures how tall the triangle is.
The base of an acute triangle is any one of its sides that we choose to use as the bottom when measuring or calculating the triangle's area. It acts like the foundation or ground line of the triangle.
SolutionThe height and base are labeled on the acute triangle.
It is important to see that the height is always the vertical line from the base to the top, forming a right angle with the base.
-
Use a formula to find the area of an acute triangle.
HintsThe formula for the area of any triangle is seen here.
$b$=base
$h$=height
In an acute triangle, when we pick a side to be the base, the height is the line that goes straight down from the vertex across from the base and meets it at a right angle.
SolutionThe equation that will result in the area of the triangle is: $A=\frac{1}{2}(12)(3)$.
base = $12\:in$
height = $3\:in$
The $8\:in$ in this triangle is not needed to find the area.
-
Find the area of a triangle using a formula.
HintsUse the formula $A=\frac{1}{2}bh$ to find the area of the acute triangle.
$b$=base
$h$=height
There are two strategies to find the area of an acute triangle:
- Find the area of the two right triangles, and then combine them for the total area.
- Find the area of the entire acute triangle.
To find the total base of this acute triangle, add $2+6$.
Solution$\begin{array}{l}A=\frac{1}{2}bh\\ b=6+2=8\\ h=4\\ A=\frac{1}{2}\left(8\right)\left(4\right)\\ A=16\ ft^2\end{array}$
-
Identify the key measurements needed to find the area of an acute triangle.
HintsIn an acute triangle, once we choose a side as the base, the height is the line that starts from the opposite vertex and drops perpendicularly to meet the base, forming a right angle.
In this triangle, two sides do not help to find the area. Which two measurements are not important for this question?
SolutionBase = $\bf{15\:cm}$
Height = $\bf{6\:cm}$
-
Solving problems with the area of an acute triangle.
HintsThe formula to find the area of an acute triangle is $A=\frac{1}{2}bh$.
$b$ = base
$h$ = height
SolutionThe area of each of these triangles is 15 square meters.
To find the area of each triangle, use the formula $A=\frac{1}{2}bh$.
$A=\frac{1}{2}(6+4)(3)=15\:m^2$
$A=\frac{1}{2}(10)(3)=15\:m^2$
$A=\frac{1}{2}(5+5)(3)=15\:m^2$
The other triangles did not have an area of $15\:m^2$
$A=\frac{1}{2}(2+3)(3)=7.5\:m^2$
$A=\frac{1}{2}(2+5)(3)=10.5\:m^2$
$A=\frac{1}{2}(3+2)(5)=12.5\:m^2$

















