Equivalent Ratios Given Parts and Wholes
Basics on the topic Equivalent Ratios Given Parts and Wholes
Equivalent Ratios
Equivalent ratios are fundamental mathematical concepts that act as the building blocks for understanding proportions and scaling in various fields. Imagine scaling a recipe or resizing an image; these everyday tasks require a solid grasp of equivalent ratios.
Understanding Equivalent Ratios
Equivalent ratios are two or more ratios that express the same relationship between quantities, despite using different numbers. They are essentially the same proportion, scaled up or down.
To understand this concept, imagine having a fruit basket with a certain number of apples and oranges. If you increase the number of fruits while maintaining the same ratio, you'll have an equivalent ratio. If there are 2 apples for every 3 oranges, doubling the quantities to 4 apples and 6 oranges still respects the original ratio, hence they are equivalent.
Here are some more examples of equivalent ratios.
| Original Ratio | Multiplier | Equivalent Ratio |
|---|---|---|
| 1:2 | 3 | 3:6 |
| 2:3 | 4 | 8:12 |
| 3:4 | 5 | 15:20 |
| 4:5 | 2 | 8:10 |
| 5:7 | 3 | 15:21 |
| 6:8 | 2 | 12:16 |
Equivalent Ratios – Example
Let's explore an example to understand how to work with equivalent ratios.
Suppose you're mixing paint and the ratio of blue to white is 3:2. If you want more paint but need to keep the color consistent, you need to increase both parts of the ratio equally.
Step 1: Identify the original ratio, which in this case is 3 parts blue to 2 parts white.
Step 2: Decide how much more paint you need. If you want to double the amount, multiply both parts of the ratio by 2.
Step 3: Calculate the new quantities. Double 3 parts blue to get 6, and double 2 parts white to get 4.
Result: The new ratio of 6:4 is equivalent to the original ratio of 3:2.
Equivalent Ratios – Guided Practice and Exercises
Let's walk through another example, imagining we're planning a party and need to adjust a punch recipe.
Now let's apply what we've learned. Consider this problem and try to solve it before checking the solution.
Equivalent Ratios – Practice
Apply what you have learned about equivalent ratios.
Mastering Equivalent Ratios – Summary
Key Learnings from this Text:
- Equivalent ratios are different expressions of the same proportional relationship.
- They can be scaled up or down by multiplying or dividing both terms by the same number.
- Understanding equivalent ratios is crucial for practical applications like cooking recipes, map reading, and creating models.
- Mastery of equivalent ratios enhances problem-solving skills and prepares students for more complex mathematical concepts.
Dive deeper into the world of ratios and proportions by checking out our interactive practice problems, videos, and printable worksheets that further explore equivalent ratios.
Mastering Equivalent Ratios – Frequently Asked Questions
Transcript Equivalent Ratios Given Parts and Wholes
Here we are at Mr. Bull's Animal Gym, where animals of all shapes and all sizes are trying to get fit. The gym's namesake and head trainer, Mr. Bull, explains a workout plan to Rachel the Rabbit, Trisha the Turtle, and Marvin the Mouse. Mr. Bull knows the importance of having both strong legs and strong arms, so his training plan includes Equivalent Ratios Given Parts and Wholes. Let's take a look at Mr. Bull's personal training plan. For part of his workout, he lifts 500 pounds with his legs. For the other part, he lifts 200 pounds with his arms. Those two parts make up the total, or the whole, of 700 to complete his workout. Mr. Bull's goal is to create workout plans for the three animals that are proportional to his workout plan. To do this, we will use the parts and the whole to calculate the constant of proportionality, represented by 'k'. The constant of proportionality will allow us to write an equation relating the variables. Once we have an equation, we will be able to solve for an unknown value with substitution. From the table, we know that legs equal 500 pounds and arms equal 200 pounds, which simplifies to 5 over 2. We can express this as a proportion, 'L' over 'a' is equal to 5 over 2. Now that we have an equation, we can calculate the proportional workout plans. The first participant is Rachel the Rabbit. She came to work out because she wants to make the Animal Olympics someday Mr. Bull looks at Rachel's arms and thinks she can work out with 9 pounds. If everything is to stay proportional, how heavy should the leg weights be? We can use the equation we just calculated to figure out the unknown part. Plug in 'A' equals 9 into the equation and then use opposite operations to isolate the 'L', multiplying both sides by 9. This gives us our missing part, 45 over 2 or 22 and a half pounds. Let's write this in the table. Now that we have the two parts, we can add those values together to find the whole. To do that you'll want to rewrite the mixed number as an improper fraction and convert the whole number 9 to a fraction with the denominator of 2. Now simplify the improper fraction to find the total, 31 and one-half pounds. Rachel knows just what to do, so Mr. Bull sends her off to get started on her workout. Now it's time for Trisha the Turtle to get her workout plan. She's come to Mr. Bull because she wants to do more than just sit around all day. He can tell from her legs that Trisha should work out with 8 and four-fifths pounds. So how much should she use to strengthen her arms? Before we calculate anything, let's convert the mixed number to an improper fraction. Now we can start calculating, using the same proportion as in our first example. But this time we plug in the value 44 fifths for 'L' and solve for 'A'. To isolate 'A', we can cross-multiply. Then, we have 88 fifths equals 5 'A'.
Using opposite operations, we divide both sides by 5, which is the same as multiplying by its reciprocal, one-fifth. Now we are left with the improper fraction 88 over 25 is equal to 'A', which is 3 and 13 twenty-fifths. Remember to fill in the chart every time you have a new value, including the total of the weights in the last column. To find this missing part, modify the equation to have the same denominator of 25, add, and then simplify to get 12 and 8 twenty-fifths. Mr. Bull sends Trisha on her way, and now it's time for the smallest animal, Marvin the Mouse. Marvin the Mouse only wandered into the gym because he thought he smelled cheese, and he has no idea what Mr. Bull has been going on about. But Mr. Bull is convinced the mouse can lift 2 and a half pounds in total. However, he's not certain about the individual leg and arm amounts. That means we know the total, 'T', but there's no 'T' in the equation we used before. What should we do? Earlier, we looked at the ratio of one part to another part, and it was constant. This time, let's look at the ratio of one part to the whole. Do we have a constant ratio? To figure it out, let's calculate the ratio of arms to total weights and check if the ratios are equivalent. For Mr. Bull's workout, the ratio of the arm weights to the total weight is simplified to 2 to 7. For Rachel the Rabbit, the ratio of the arm weights to the total weight also simplifies to 2 to 7. And for Trisha, it's the same. This confirms that all of our previous calculations are correct, since the ratio is 2 to 7 for all the workouts.
We can then write that A over T equals 2 over 7. To isolate the 'A', we can multiply both sides by 'T' to get 'A' equals 2 over 7 times 'T'. Now, that we have our equation, we can plug in two and a half, or 5 halves, for 'T' and solve to get 'A' equals 5/7. So, we know the amount of arm weights, but how can we figure out the amount of the leg weights? Just subtract the arm weights from the total weight. The mouse should lift leg weights equal to 1 and eleven fourteenths pounds. While Trisha and Rachel are busy lifting weights, let's summarize. When considering proportional relationships with parts and wholes, be aware they can be written in different ways. We can compare the two parts, or we can compare a part to the whole. Also, the numbers can be whole numbers or fractions. But no matter what, the process remains the same. Marvin the Mouse didn't even start his workout, Rachel the Rabbit finished hers a short while ago. and Mr. Bull has closed up shop for the day, but it looks like Trisha the Turtle will be a little while.
Equivalent Ratios Given Parts and Wholes exercise
-
Given a ratio, find an equivalent ratio.
HintsStart by setting up a ratio of $a$ to $b$. This represents the constant of proportionality, $k$.
The ratio of workout days to rest days is $\frac{5}{1}$.
SolutionAccording to Mr. Bull, the best workout plan in a month with 30 total days, there should be 25 workout days, and 5 rest days.
$\frac{25}{5}=\frac{5}{1}$
$25+5=30$
-
Apply the process needed to complete the ratio table.
HintsThe unknown variable in an equation is the variable that you are solving for.
Always simplify the constant of proportionality.
If the constant of proportionality is $\frac{350}{150}$, simplify by dividing both numbers by the greatest common factor. In this case, the GCF is $50$ and $\frac{350\div 50}{150\div 50}=\frac{7}{3}$.
SolutionUse the values in the table to create a ratio representing Mr. Bull's leg and arm workout: $k=\frac{300}{200}$ and then simplify the ratio to: $\bf{\frac{3}{2}}$
Using the variables $L$ for legs and $A$ for arms, we can then identify the equation: $\frac{L}{A}=\frac{3}{2}$.
For Scarlett's workout plan, Mr. Bull already knows that he wants her leg workout to include $4\frac{1}{2}$ lbs, which can be written as an improper number: $\bf{\frac{9}{2}}$
Since Mr. Bull wants to make her workout proportional to his, he will use the same equation, $\frac{L}{A}=\frac{3}{2}$, and the unknown variable is $\bf{A}$ (arms).
$\dfrac{\frac{9}{2}}{A}=\dfrac{3}{2}$
Cross multiplication can be used to find the missing variable of $A$.
$\begin{array}{l}\frac{\frac{9}{2}}{A}=\frac{3}{2}\\ \\ 3\left(A\right)=\frac{9}{2}\left(2\right)\\ \\ \frac{3A}{3}=\frac{9}{3}\end{array}$
$A$=$\bf{3}$ lbs
Scarlett's total workout is:
$L+A=4\frac{1}{2}+3$ = $\bf{7\frac{1}{2}}$ lbs
-
Find the proportional equation and use it to solve.
HintsThe word problem represents a proportional equation where the constant of proportionality, $k$, is equal to the ratio of water to pots, $\frac{W}{P}$, or pots to water, $\frac{P}{W}$.
To find the constant of proportionality, $k$, simplify the fraction of $\frac{W}{P}$ or $\frac{P}{W}$.
To solve a proportional equation, use opposite operations or cross multiply.
There are a total of three true statements.
SolutionThe word problem represents a proportional equation where the constant of proportionality, $k$, is equal to the ratio of water to pots, $\frac{W}{P}$, or pots to water, $\frac{P}{W}$.
- $\frac{W}{P}=k$ or $\frac{P}{W}=k$
- $\frac{W}{P}=\frac{8}{32}$ or $\frac{P}{W}=\frac{32}{8}$
- $\frac{W}{P}=\frac{1}{4}$ or $\frac{P}{W}=\frac{4}{1}$
- $\frac{23}{P}=\frac{1}{4}$
- $P\cdot 1=23\cdot 4$
- $P=92$
-
Understand how a ratio table works.
HintsWrite an equation by setting the constant of proportionality, $k$, equal to the ratio of bananas to strawberries, $\frac{B}{S}$.
When solving for an unknown, cross multiply or use opposite operations.
When adding fractions, make sure both fractions have a common denominator, then add the numerators.
- Example: $2\frac{3}{7}+4\frac{5}{7}=6\frac{8}{7}=7\frac{1}{7}$
SolutionMr. Bull
- Use the given information to fill in the first two columns in the table.
- Add bananas and strawberries together to find the total: $4+12=16$
- Find the constant of proportionality, $k$, and write an equation using the ratio of bananas to strawberries.
- $k=\frac{4}{12}=\frac{1}{3}$
- Since $k=\frac{B}{S}$, the equation is $\frac{B}{S}=\frac{1}{3}$.
- Since we know that there are $3\frac{1}{4}$ bananas in Rachel's smoothie, we can substitute $B$ into the equation.
- First, convert $3\frac{1}{4}$ into an improper fraction, $\frac{13}{4}$.
- $\frac{\frac{13}{4}}{S}=\frac{1}{3}$
- Cross multiply to solve for $S$.
- $S\cdot 1=\frac{13}{4}\cdot \frac{3}{1}$
- $S=\frac{39}{4}=9\frac{3}{4}$
- To find the total add bananas and strawberries: $3\frac{1}{4}+9\frac{3}{4}=12\frac{4}{4}=13$
- Convert $5\frac{1}{4}$ into an improper fraction, $\frac{21}{4}$, then substitute for $S$ in the equation, $\frac{B}{S}=\frac{1}{3}$.
- $\frac{B}{\frac{21}{4}}=\frac{1}{3}$
- Use opposite operations and multiply both sides by $\frac{21}{4}$.
- $B=\frac{1}{3}\cdot \frac{21}{4}=\frac{21}{12}=1\frac{9}{12}=1\frac{3}{4}$
- To find the total, add bananas and strawberries: $1\frac{3}{4}+5\frac{1}{4}=6\frac{4}{4}=7$
- Since we only know the total, create a new equation using a new ratio, $\frac{\text{Strawberries}}{\text{Total}}$.
- We can use any of the numbers in the table, but the easiest ratio can be found from using Mr. Bull's numbers: $\frac{S}{T}=\frac{12}{16}$
- The new equation is, $\frac{S}{T}=\frac{3}{4}$.
- Convert Martin's total, $6\frac{2}{5}$, into an improper fraction, $\frac{32}{5}$, and substitute for $T$ in the equation.
- $\frac{S}{\frac{32}{5}}=\frac{3}{4}$
- Use opposite operations and multiply both sides by $\frac{32}{5}$ to isolate $S$.
- $S=\frac{3}{4}\cdot \frac{32}{5}=\frac{96}{20}=\frac{24}{5}=4\frac{4}{5}$
- To find the number of bananas, subtract strawberries from the total: $6\frac{2}{5}-4\frac{4}{5}=5\frac{7}{5}-4\frac{4}{5}=1\frac{3}{5}$
-
Understanding equivalent ratios to find a ratio in simplest form.
HintsRemember, a ratio compares the values of two different quantities. For example if you were making lemonade, you may use a ratio comparing the number of lemons to the cups of sugar.
Example: 8 lemons for every 1 cup of sugar
Ratios can be written in three different ways:
- $8$ to $1$
- $\frac{8}{1}$
- $8:1$
Equivalent ratios can be found by multiplying or dividing a ratio by a number to scale it up or scale it down.
For example, if we were to follow the recipe of $8$ lemons for every $1$ cup of sugar, but we wanted more lemonade, we can multiply the numbers by $3$ to find an equivalent ratio of $\frac{24}{3}$.
If we had a ratio of $\frac{10}{5}$ we can divide both the numerator and denominator by $5$ to simplify the ratio to it's lowest terms.
$\frac{10}{5}=\frac{2}{1}$
SolutionFor every 2 bananas, there is 1 tablespoon of peanut butter.
The ratios in the table can all be simplified to $\frac{2}{1}$.
-
Decide which model represents the ratio table.
HintsFind the constant of proportionality, $k$, by setting up a ratio and simplifying.
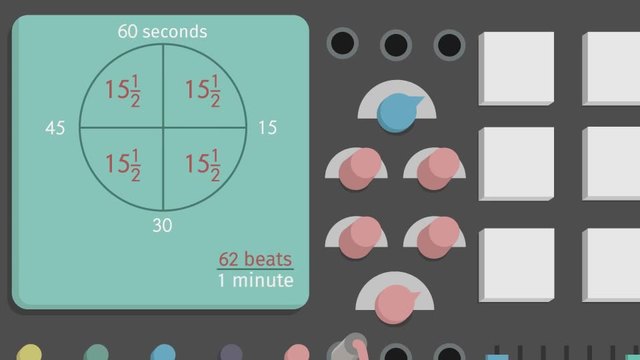
The shaded portion in tape diagrams and circle models represents the part, and the entire diagram represents the whole.
In a double number line, the top line represents the part and the bottom line represents the whole.
SolutionTable 1: $\begin{array}{|c|c|} \hline \\ 3\frac{1}{3} & \frac{20}{21}\\ \\ \hline \\ 4 & \frac{8}{7}\\ \\ \hline \\ 4\frac{1}{2} & 1\frac{2}{7}\\ \\ \hline \end{array}$
- To find the constant of proportionality, $k$, set up a ratio between two numbers in any row of the ratio table.
- Using the first row of numbers, the constant of proportionality is, $k=\frac{3\frac{1}{3}}{\frac{20}{21}}=\frac{\frac{10}{3}}{\frac{20}{21}}=\frac{10}{3}\cdot \frac{21}{20}=\frac{210}{60}=\frac{7}{2}$
- The visual representation that shows the constant of proportionality, $k=\frac{7}{2}$, is the tape diagram.
- This is because $\frac{7}{2}$ written as a mixed number is $3\frac{1}{2}$, and $3$ and$\frac{1}{2}$ boxes are shaded in.
- To find the constant of proportionality, $k$, set up a ratio between two numbers in any row of the ratio table.
- Using the second row of numbers, the constant of proportionality is, $k=\frac{1\frac{1}{2}}{3}=\frac{\frac{3}{2}}{3}=\frac{3}{2}\cdot \frac{1}{3}=\frac{3}{6}=\frac{1}{2}$.
- The visual representation that shows the constant of proportionality, $k=\frac{1}{2}$, is the double number line
- This is because the last number on the top line, $50$, is the part when the whole is $100$, and $\frac{50}{100}=\frac{1}{2}$.
- To find the constant of proportionality, $k$, set up a ratio between two numbers in any row of the ratio table.
- Using the third row of numbers, the constant of proportionality is $k=\frac{2\frac{3}{4}}{2\frac{1}{5}}=\frac{\frac{11}{4}}{\frac{11}{5}}=\frac{11}{4}\cdot \frac{5}{11}=\frac{55}{44}=\frac{5}{4}$
- The visual representation that shows the constant of proportionality, $k=\frac{5}{4}$, is the fraction $\frac{15}{12}$.
- This is because dividing both numbers in the fraction, $\frac{15}{12}$, by the greatest common factor of $3$ yields $\frac{5}{4}$.
- To find the constant of proportionality, $k$, set up a ratio between two numbers in any row of the ratio table.
- Using the first row of numbers, the constant of proportionality is $k=\frac{2\frac{1}{3}}{7}=\frac{\frac{7}{3}}{7}=\frac{7}{3}\cdot \frac{1}{7}=\frac{7}{21}=\frac{1}{3}$
- The visual representation that shows the constant of proportionality, $k=\frac{1}{3}$, is the circle model.
- This is because $2$ of the $6$ pieces are shaded which yields the fraction $\frac{2}{6}=\frac{1}{3}$.