Changing Scales

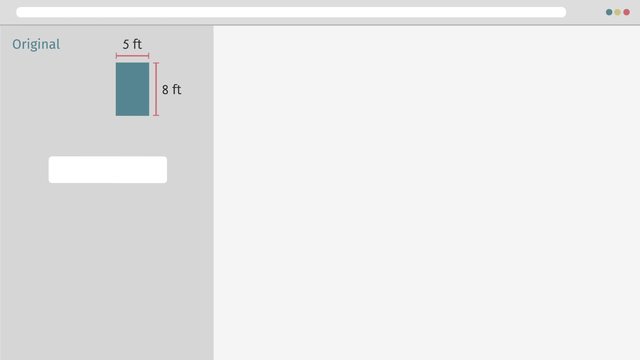
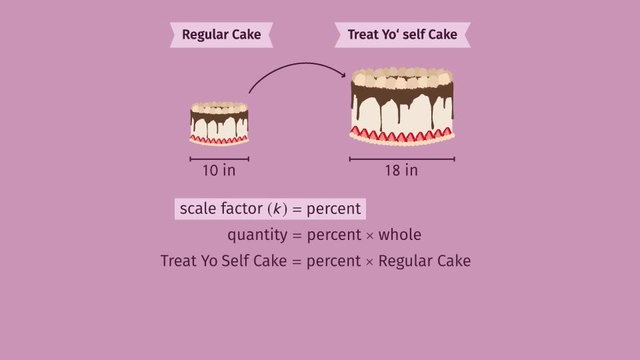
This year, King Wallace is resolving to cut down on junk food. But before he starts his New Year's diet he'll treat himself to one last cake. To help manage his portion size, King Wallace summons his court magician, Mr. Magic who knows all about changing scales. This is King Wallace's last cake before his big diet, so he should really treat himself. He asks Mr. Magic to pump up the size of his cake just this once! The regular cake is 10 inches in diameter but King Wallace wants an all-out 18-inch treat yo'self cake! What percent should Mr. Magic use as a scale factor in order to enlarge his cake? To find out, let's use the familiar equation, quantity equals percent times whole. We can adapt this familiar equation so that 'percent' is our scale factor quantity is any length on our scaled object and the whole is the corresponding length on the original object. Of course, these aren't just any objects, but a regular cake and an enlarged "Treat Yo'Self" cake! Now that we know all our variables, let's substitute in actual values to complete our equation. King Wallace's regular cake starts out measuring 10 inches in diameter -- that's the original length. When we multiply this length by the percent, or scale factor, we get the 18-inch diameter of the enlarged Treat Yo'Self Cake. Now to solve for the percent, we just divide both sides by 10 inches giving us 1.8 which means the diameter of the scaled cake is 180% larger than the original. So we know that this is a true enlargement because our scale factor is greater than 1. That's a whole cakeload of calories! King Wallace immediately regrets ordering such a gigantic cake. What about his New Year's resolution? What about his beach body? So, King Wallace asks Mr. Magic to reverse his scaling, and fast. What scale factor should Mr. Magic use to reverse his magic and turn the Treat Yo' Self cake back into a Regular cake? Let's set up our equation again. But which cake is the original now, and which is the scaled version? It all depends on the direction of the transformation. Because we're starting off with the 18-inch Treat Yo'Self Cake, that's our original making the 10-inch Regular cake the scaled version. Using division to solve for the percent we see that Mr. Magic should use a scale factor of about 55.6% to restore the cake to its original size. 55.6% is less than 1, which makes this a reduction. Note that the scale factor for the enlargement and the scale factor for the reduction are actually reciprocals of each other in fraction form. Makes sense, because all we did is flip the direction of the transformation, not change the numbers we were working with. Now that the cake is back to regular size, King Wallace decides he really shouldn't even eat THAT much cake. Maybe just a small sample a teeny tiny taste of cake will satisfy his cravings. Mr. Magic, it's time to make a Mini cake! What percent should Mr. Magic use as a scale factor to reduce the 10-inch Regular cake to a 4-inch Mini cake? Let's set up the equation just like before. The 10-inch cake is the original now and the 4-inch cake is the scaled version. That makes the scale factor 40%. If we wanted to reverse the direction of the transformation, how could you quickly find that scale factor? Just take the reciprocal of 40%. That's 10 fourths or 250%. King Wallace is disappointed with his Mini cake. It's just so tiny. If only he could have a few more bites. King Wallace is tired of denying himself! The diet starts tomorrow! He's going all-in for the Treat Yo'Self Cake! What percent should Mr. Magic use to transform the Mini cake into an enlarged Treat Yo'Self Cake? Time for our trusty formula, but oh no! Mr. Magic has done so many transformations, he's fogotten the diameters of all the cakes! How can we find the missing scale factor, using only the information we have in the diagram? Well, we know the Treat Yo'Self Cake is 180% of the size of the Regular Cake. And the Mini Cake is 40% the size of the Regular Cake. Dividing both sides of the equation by the diameter of the Regular Cake leaves us with an equation we can work with. From here, we can solve. 180% divided by 40% equals 450% the scale factor from the Mini Cake to the Treat Yo'Self Cake. While King Wallace is savoring his delicious cake, let's review. Scale factors tell us the constant of proportionality between two similar objects. We can determine the scale factor by using the equation quantity equals percent times whole, where the whole is the original object, and quantity is the scaled version of that object. The scale factor depends on the direction of the transformation. Scale factors over 100% result in enlargements and less than 100% result in reductions. And to reverse a scaling, you can just use the reciprocal of your scale factor. So magical transformations can always be undone. It looks like King Wallace has truly treated His Royal Self! You want something to wash that down, Your Highness? Oh, smart thinking.