Area of Composite Shapes

Basics on the topic Area of Composite Shapes
In This Video on Area of Composite Shapes
Zuri and Freddie are architects for the day, and want to build a tall building. However, they want to make sure the building isn’t too big, so need to calculate the area of the composite shape! In the end, we see that all isn’t as it appeared to be… At the bottom of this page is a finding area of composite shapes worksheet.
How to Find the Area of Composite Shapes
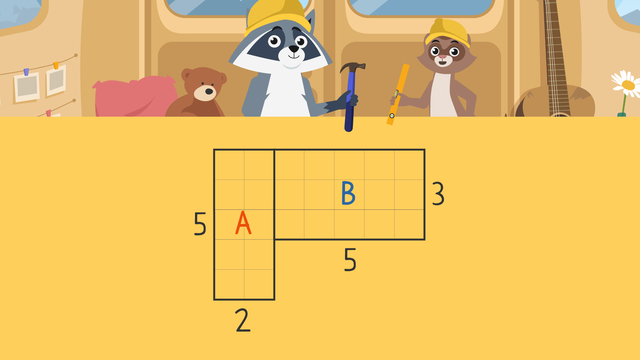
First, decompose the shape into two rectangles.
Then, find the area of the two shapes, using the formula area equals length times width.
Next, add together the area of the two rectangles.
Finally, add the unit of measurement.
For more practice, use the worksheet area of composite shapes below.
Below this area of composite shapes video you will find an area of composite shapes worksheet. You will also find area of composite shapes practice too!
Transcript Area of Composite Shapes
Freddie and Zuri are ready to be architects for the day! They want to ensure that the area of the building isn't too big, but the floor plan is a composite shape! Let's learn all about how to find the area of composite shapes. A composite shape is a figure that consists of two or more shapes put together. To find the area of a composite shape, first, decompose, or break down, the composite shape into regular shapes, like rectangles. Then, find the area of each individual rectangle using area equals length multiplied by width. Finally, add together the area of all decomposed shapes to find the total area of the composite shape, adding the unit of measurement needed. Let's practice before we help Zuri and Freddie. First, decompose the shape. This shape can be decomposed two ways to make two regular shapes. For this shape, let's separate it here. There are now two rectangles which we can label A and B. Next, find the area of both rectangles. Starting with rectangle A, use length times width to solve for area. When shapes are decomposed, use only the measurements that cover its full length and width. Use five for length and two for width! Five times two equals ten, making the area of rectangle A ten square units. Now solve rectangle B. Use five for length and three for width. Five times three equals fifteen, so rectangle B has an area of fifteen square units. Finally, add together the area of rectangle A and rectangle B to find the area of the entire composite shape. Ten plus fifteen equals twenty-five. The composite shape has an area of twenty-five square units. Check the answer by counting all the unit squares inside the composite shape, which is twenty-five. Now we have looked at finding the area of a composite shape, let's help Zuri and Freddie calculate the area of the building using the floor plan. What is the first step? Decompose the composite shape. Let's separate it here, like this. We have a rectangle and a square, so label them A and B. What is the next step? Find the area of both shapes, starting with shape A. Remember, we can find the area multiplying length by width. What is the length and width of shape A? It is four and two. Now solve the equation. What is four times two? Eight, so the area of shape A is eight square units. Now, let's find the area of shape B. What is the length and width of shape B? It is six and six. Now solve the equation. What is six times six? Thirty-six, so the area of shape B is thirty-six square units. What is the final step? Add together the are of shape A and shape B to find the area of the composite shape. What is eight plus thirty-six? It is forty-four, so the composite shape has an area of forty-four square units. Check the answer by counting all the unit squares inside the composite shape, which is forty-four. Zuri and Freddie decide this is the perfect size, so while they construct the building, let's review! Remember, to find the area of a composite shape, first, decompose, or break down, the composite shape into regular shapes, like rectangles and use only the measurements that cover the decomposed shapes full length and width. Then, find the area of each individual shape. Finally, add together the area of all decomposed shapes, to find the total area of the composite shape. Check your work by counting all the unit squares inside the composite shape. "Phew, that sure was a lot of hard work to build." "It was, but it looks magnificent now!" "Well, I guess we should decompose this now we are finished!"
Area of Composite Shapes exercise
-
Help Zuri and Freddie!
HintsFind the area of the decomposed shapes by multiplying the length by width.
Then, add together the area of each decomposed shape to find the total area.
Remember, you can check your work by counting the unit squares inside the shapes.
SolutionFirst, find the area of shape A: 4 x 2 = 8
Then, find the area of shape B: 6 x 6 = 36
Finally, add the area shape A and shape B together to find the total area of the composite shape.
8 + 36 = 44 Square units
-
Are these shapes correctly decomposed?
HintsRemember, we try to decompose shapes into rectangles to make it easier to find the area.
A shape can be partitioned into more than two parts.
SolutionAlways decompose the shape into smaller rectangles.
Since two shapes were correctly decomposed by Zuri and Freddie, they go into the yes category.
Two shapes were not correctly decomposed by Zuri and Freddie because they are not decomposed into smaller rectangles. These two shapes go in the no category.
-
What is the area of this composite shape?
HintsYou can decompose the shape to calculate the area of each shape, then add them together for the area.
Try decomposing the shape like this to help you find the area.
Remember to find the area you multiply the length by the width.
Remember, you can check your work by counting the unit squares inside the shapes.
SolutionDecompose the composite shape into two shapes.
To find the area of shape A, solve 5 x 2, which is 10.
To find the area of shape B, solve 5 x 3, which is 15.
Next, add the area of both shapes together. 10 + 15 = 25.
Finally, add the unit of measurement.
The answer is: 25 square units.
-
Find the area of the composite shape.
HintsSolve the area of shape A and shape B first.
To find the area of decomposed shapes, multiply the length by the width.
After you have found the area of shape A and shape B, add them together to find the area of the composite shape.
SolutionFirst, find the area of shape A: 7 x 4 = 28 square units
Next, find the area of shape B: 3 x 4 = 12 square units
Finally, add the area of both shapes to find the total area of the composite shape: 28 + 12 = 40 square units
-
What is the correct order of steps for finding the area of composite shapes?
HintsRemember, always start by breaking down the shape.
Solution- Decompose the shape into multiple shapes.
- Find the area of each shape.
- Find the sum of all the areas.
- Add the correct unit of measurement.
-
Solve Zuri and Freddie's problem!
HintsFind the area of each floor plan first, then compare to see which one has the bigger area.
Remember, solve shape A and shape B, then add them together to find the total area of the composite shape.
SolutionFind the area of Zuri's floor plan.
Shape A: 9 x 6 = 54 square units
Shape B: 3 x 3 = 9 square units
Total area: 54 + 9 = 63 square units
Find the area of Freddie's floor plan.
Shape A: 8 x 7 = 56 square units
Shape B: 3 x 2 = 6 square units
Total area: 56 + 6 = 62 square units
Now compare the total area of both floor plans. Zuri's floor plan is 63 square units, and Freddie's floor plan is 62 square units. Since 63 is greater than 62, they will build Zuri's floor plan since it has a bigger area than Freddie's floor plan.