Area of a Shape
- Measuring the Area of a Shape
- The Area of a Shape – Definition
- Units Square – Definition
- Counting Unit Squares
- Square Units – Definition
- Finding the Area of Shapes in Square Units
- Finding the Area of Irregular Shapes
- Area – Examples
- The Area of Shapes – Summary of Steps
- Frequently Asked Questions concerning The Area of a Shape

Basics on the topic Area of a Shape
Measuring the Area of a Shape
Sometimes you need to determine the size of a shape. The size, over which an area spreads out is called the area. This text offers the necessary knowledge on how to find the area of a shape by counting unit squares.
The Area of a Shape – Definition
Let’s learn more about finding the area of a shape and determining the area with square units in 3rd grade. The area of a shape is the amount of space it takes up on a flat surface. Area is always measured in square units.
Units Square – Definition
A unit square is a special square that measures one unit in length on each side and thus has an area of one square unit. Unit squares are used to determine area in square units.
We define unit squares as taking up one square unit of area. We can lay unit squares side by side, without spaces or overlaps, across the entire surface of a figure. This helps us measure the amount of space, or area, the figure takes up.
Counting Unit Squares
Count the number of square units needed to cover a surface one at a time. This will calculate the total area and is why the area is measured in square units.
Square Units – Definition
Let’s explain the relationship between area and square units: square units are used to measure area. This is why questions ask you to find the area in square units and why is the area labeled with square units.
The definition of square units is a quantity of measure used to determine the amount of space a figure takes up on a flat surface. The area of a shape is expressed in square units. How would you calculate square units? Let’s say a shape is covered by 40 unit squares, you would say its area measures 40 square units.
Finding the Area of Shapes in Square Units
Finding the area of a shape is simple. After laying unit squares across the entire surface of a figure, count each square. For example, you can draw unit squares across the entire surface to show the area of the rectangle. This is how to find the total area of a shape. Finally, label the area of a figure in square units.
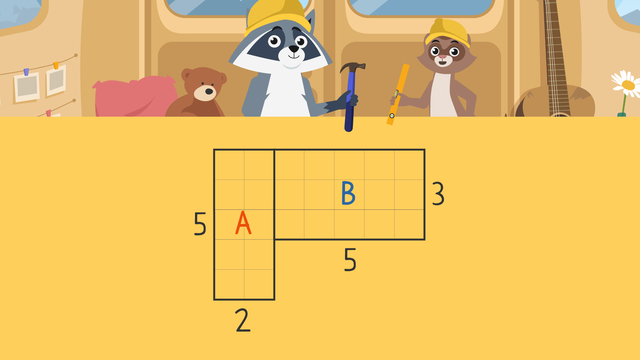
Finding the Area of Irregular Shapes
Finding the area of an odd shape is the same. After laying unit squares side by side, across the entire surface of the irregular figure, count each square.
Area – Examples
Below are examples of area. To find the area of each figure in square units,simply count the unit squares that cover each.
Comparing the area of irregular shapes and regular shapes.
Comparing the area of two irregular shapes.
The Area of Shapes – Summary of Steps
| Step # | What to do |
|---|---|
| 1 | Lay unit squares across the entire surface of the figure. |
| 2 | Count each square. |
| 3 | Label the area of the figure in square units. |
Have you practiced yet? On this website, you can also find worksheets on area in square units and exercises.
Frequently Asked Questions concerning The Area of a Shape
Transcript Area of a Shape
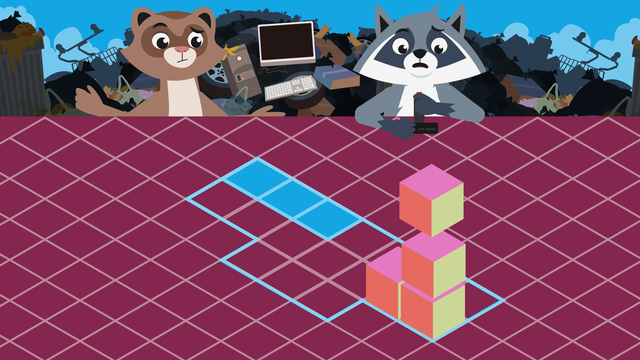
Zuri and Freddie have been playing BlockBuilders on an old computer they found in the landfill. After building with blocks, they are curious to know who's world is larger. In order to determine which structures are larger, they will need to calculate their area or the size of the surface. Let's help them measure the area using unit squares. "Area of a Shape Using Unit Squares" Area is the amount of space a figure takes up on a flat surface. We can determine the area of a figure using unit squares. Unit squares measure one unit in length on each side. This means the space they take up measures one square unit of area. We can lay unit squares side by side, without spaces or overlaps, across the entire surface of a figure. This measures the amount of space it takes up. To calculate the total area, you need to count each unit square. We can use this same strategy to determine the area for Zuri and Freddie's builds. They want to compare the areas of the houses, pools and playgrounds they built. Let's begin by counting all the unit squares within the floor plan of Zuri's house. We start in one corner and carefully count each square: one, two, three, four, five, six, seven, eight, nine, ten, eleven, twelve, thirteen, fourteen, fifteen, sixteen, seventeen, eighteen, nineteen, twenty! Zuri's house is twenty square units. Let's count the floor plan of Freddie's house now. Ready? One, two, three, four, five, six, seven, eight, nine, ten, eleven, twelve, thirteen, fourteen, fifteen, sixteen, seventeen! Freddie's house is seventeen square units. So, the area of his house is a little smaller than Zuri's. Now let's calculate the areas of their pools! How do we determine the area of Zuri's pool? We count all the unit squares within the figure. Ready? One, two, three, four, five, six, seven, eight, nine, ten, eleven, twelve, thirteen, fourteen. Zuri's pool is fourteen square units. How do we determine the area of Freddie's pool? We count all the unit squares within the rectangle. Let's count: one, two, three, four, five, six, seven, eight, nine, ten, eleven, twelve, thirteen, fourteen, fifteen, sixteen, seventeen, eighteen, nineteen, twenty, twenty-one, twenty-two, twenty-three, twenty-four! Freddie's pool is twenty-four square units. So, Freddie has the larger pool. Finally, they want to compare the area of their playgrounds. How do we determine the area of Zuri's playground? We count all the unit squares within the floorplan. Ready? One, two, three, four, five, six, seven, eight, nine, ten, eleven, twelve, thirteen, fourteen! The area of Zuri's playground measures fourteen square units! How do we determine the area of Freddie's playground? We count all the unit squares within the figure. Ready? One, two, three, four, five, six, seven, eight, nine, ten, eleven, twelve, thirteen, fourteen. Freddie's playground also measures fourteen square units. Even though their playgrounds are different shapes, they still have the same area! Before Zuri and Freddie compare their worlds, let's review! Remember, area is the amount of space a figure takes up on a flat surface. We can find the area of a figure using unit squares. These are boxes that measure one unit of length on each side and have an area of one square unit. We lay the unit squares side by side without spaces or overlaps across the entire surface of the figure. Then, we count all the squares to find the area. Finally, we write our total area in square units. "Hmmm, so who do you think made bigger stuff?" "Well it looks like…hey, what happened?!" "Bummer! I guess we'll have to start all over. " “But, we can work together this time!"
Area of a Shape exercise
-
What is the area of Zuri's new house?
HintsDid you count each square?
In this example, the area would be 8 square units.
SolutionThe answer is 18 square units.
Zuri's house covers 18 squares on a flat surface, therefore the area is 18 square units.
-
Can you assign the area of the builds correctly?
HintsRemember to count each square once to find the total square units.
This example shows an area of 15 square units.
SolutionHere is an example of each of the areas.
Remember to count each square once to find the total number of square units and therefore the total area.
-
Can you find the areas of Freddie and Zuri's creations?
HintsRemember, each square unit = 1.
Remember to count each square only once. You could start by counting along the top line then moving down.
This example is made up of 10 square units.
SolutionThe answer to the first problem is 12.
If you count each square unit you will reach the answer 12.
The area highlighted is made up of 12 square units.
__________________________________________________________________
- The second area is made up of 25 square units.
- The third area is made up of 20 square units.
- The fourth area is made up of 17 square units.
- The fifth area is made up of 14 square units.
- The sixth area is made up of 31 square units.
-
Can you order the areas?
HintsAs you are ordering, compare each area with the one above and below it.
Remember to put the build with the smallest area first.
This example is made up of 9 square units.
SolutionThe above image shows the smallest area and the largest area.
The smallest area is made up of 13 square units and the largest area is made up of 21 square units.
From smallest to largest:
- 13 square units
- 14 square units
- 17 square units
- 19 square units
- 21 square units
-
Find the correct area.
HintsRemember to count each square once to find the area.
This example is made up of 4 square units.
SolutionThis is the correct area made up of 16 square units.
-
Complete the areas of each build.
HintsStart by counting the light blue squares then think about how many more you need to complete the total area.
Once you have highlighted, check the total area of each block build.
Solution- The first area has a total of 18 square units. There are already 14 square units, so 4 extra square units needed highlighting. 18 = 14 + 4.
- The second area has a total of 22 square units. There are already 17 square units, so 5 extra square units needed highlighting. 22 = 17 + 5.
- The third area has a total of 28 square units. There are already 22 square units, so 6 extra square units needed highlighting. 28 = 22 + 6.
- The fourth area has a total of 25 square units. There are already 18 square units, so 7 extra square units needed highlighting. 25 = 18 + 7.