Applying Algebraic Properties of Equality


Basics on the topic Applying Algebraic Properties of Equality
After this lesson, you will be able to rewrite and modify equations by applying the different properties of equality.
The lesson begins by teaching you how to write an equation representing a real-life problem and to apply the addition property of equality to rewrite it. It leads you to learn how to combine like terms on the same side of the equation and isolate the variable used in the equation by applying the subtraction property of equality. It concludes with determining the solution of the equation by applying the other properties of equality such as the associative properties of multiplication and addition and the commutative property of addition.
Learn about rewriting an equation using the different properties of equality by helping the flobbit Wilbo compute for the length of time that he shall spend on napping so he could complete all his tasks for the day!
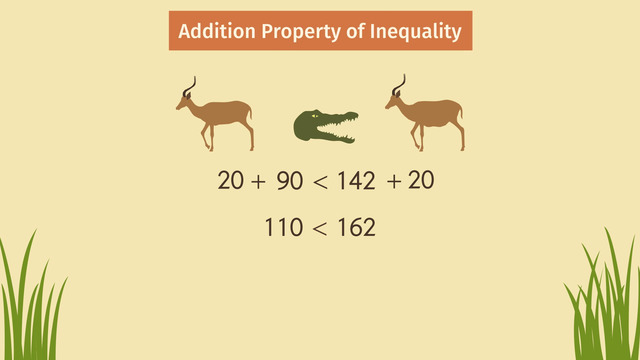
This video introduces new concepts, notation, and vocabulary such as the Addition Property (adding equal quantities to both sides of the equation will retain the equality of the two sides); Subtraction Property (subtracting equal quantities from both sides of the equation will retain the equality of the two sides); Associative Property of Multiplication (given a multiplication operation involving more than two factors, the order in which factors are grouped will not affect the product); Associative Property of Addition (given an addition problem involving more than two addends, the order in which addends are grouped will not affect the sum; and Commutative Property of Addition (the order of the addends in an addition problem will not affect the sum).
Before watching this video, you should already be familiar with variables, like terms, equations, and writing an equation to represent a word problem.
After watching this video, you will be prepared to learn how to solve more word problems involving equations such as distance, age, and unknown angle word problems algebraically.
Common Core Standard(s) in focus: 7.EE.B.3 and 7.EE.B.4 A video intended for math students in the 7th grade Recommended for students who are 12-13 years old
Transcript Applying Algebraic Properties of Equality
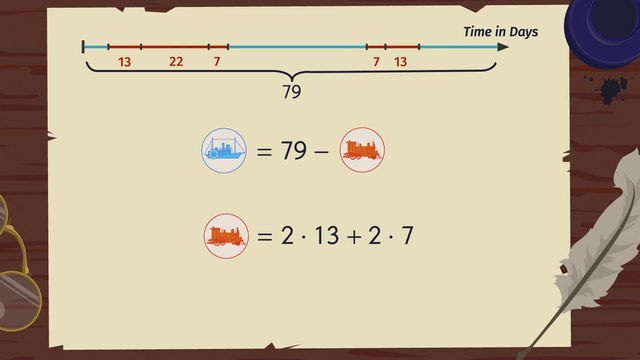
In this house, there lives a flobbit named Wilbo. He's still sleeping, as flobbits tend to do but it’s time to get up now ‘cause there's a lot to do. Today, Wilbo has to take care of his pumpkins, eat delicious mushrooms with his friends and go hiking in the Flobbit-mountains. Sounds like a long day. To figure out how long, we're going to need to Apply Algebraic Properties of Equality. Wilbo plans to spend 210 minutes taking care of his pumpkins and he needs 40 minutes for lunch. Wilbo plans to go hiking for one fourth of the day and the rest of the day if there is time left, he wants to take a nap. For taking care of the pumpkins and going hiking, he plans to spend a total time of 330 minutes. So how long, in minutes, will his day be? How can we solve this problem using math? Of course, first we have to set up the equation we want to solve. Let's substitute one quarter of a day for hiking and let's let 'd' stand for the number of minutes in a Flobbit day we can also substitute 210 for the time it takes Wilbo to care for his pumpkin patch. Oops! We almost forgot to give Wilbo time for lunch! We have to add 40 minutes to the equation, but where? Since this is an equation, you have to add 40 on both sides of the equation to keep it equal. This is the Addition Property for equations. It says that, if you add a value to one side of the equation, you also have to add it to the other side to keep the equation equal. The new equation, including time for Wilbo's lunch, is: one fourth 'd' plus 250 equals 370. Let's review the subtraction property. It's like the addition property, but involves subtraction instead. If you subtract something from one side of the equation, you must subtract the same amount from the other side to keep the equation equal. Here, we apply the subtraction property to isolate the variable 'd'. If a variable is multiplied by a fraction, that's the same as multiplying the variable by the numerator and dividing by the denominator. When we see something like this, we can simply multiply by the reciprocal of the fraction to isolate the variable. In this case, we multiply both sides by 4 over 1. The Associative Property of multiplication says: if you're multiplying several numbers together, the order in which the numbers are grouped does not matter. In case you were wondering, there's an associative property of addition as well. Getting back to our equation, Wilbo's work day is 480 minutes, or 8 hours long. Since there are 60 minutes in each hour, we can convert 480 minutes to hours by multiplying our total by the number of minutes per hour to find out how long Wilbo has to work. So, how much time is left for Wilbo's all-important nap? Let's see what we've got: Wilbo wants his day, which is 480 minutes long, to be split up between 4 very important tasks. He wants to take care of his pumpkins, eat lunch, go hiking, and then take a nap. If we let 'p' stand for Wilbo's pumpkin care time and 'l' stand for lunch 'h' for hiking and 'n' for time spent napping we can rewrite the equation like this: But does the order in which Wilbo completes his tasks matter? Couldn't he go hiking, take a nap, eat lunch, and then care for his pumpkins and it would take the same time? As long as the signs remain the same, the order in which you add does not matter. This is called the Commutative Property of Addition. Commute just means 'to move'. Your parents might commute to work in the morning... Back to our problem. Before, we figured out that Wilbo has 370 minutes planned for pumpkin patch maintenance, lunch, and hiking. Replacing this in our equation, we can see this problem's looking easier already! Notice we have addition with 'n'. If we have addition and want to isolate the variable, we need to use its opposite operation, subtraction, on both sides of the equal sign. Subtracting the two numbers on the right side of the equation we see that Wilbo can take a nap for 110 minutes.
Applying Algebraic Properties of Equality exercise
-
Explain the addition and subtraction properties of equality.
HintsTo understand the definition of the addition property of equality, if $a=b$ then $a+c=b+c$:
- Let $a=2$, $b=2$ and $c=1$.
- Since $2=2$ then $2+1=2+1$. The equation is still balanced.
- Let $a=2$, $b=2$ and $c=1$.
- Since $2=2$, then $2-1=2-1$. The equation is still balanced.
If we are given the equation $3d+5=10$ and want to add $1$, we add $1$ to both sides to keep it equal. This is called the addition property of equality.
- $3d+5+1=10+1$
If we are given the equation $3d+5=10$ and want to isolate the variable $d$, we first subtract $5$ from both sides. This is called the subtraction property of equality.
- $3d+5-5=10-5$
Solution1. Addition Property of Equality
- If $a=b$ then $a+c=b+c$.
- This statement means that to keep an equation equal, you have to add the same number to both sides of the equation.
- In Wilbo's equation, $ \frac{1}{4}d+210$ = $330$, to add 40 minutes for lunch into the equation, Wilbo needs to add it to the left and right side of the equation to keep the equation equal.
- $ \frac{1}{4}d+210+40$ = $330+40$
- Combine like terms: $ \frac{1}{4}d+250=370$
- Therefore, Wilbo used the addition property of equality to add lunch into his equation.
- If $a=b$ then $a-c=b-c$
- This statement means that to keep an equation equal, you have to subtract the same number from both sides of the equation.
- In Wilbo's equation $ \frac{1}{4}d+250=370$, in order to isolate $d$, he needs to subtract 250 from both sides of the equation. This keeps the equation equal.
- $ \frac{1}{4}d+250-250=370-250$
- Combine like terms: $ \frac{1}{4}d=120$
- Therefore, Wilbo used the subtraction property of equality to isolate the variable $d$.
-
Match the properties of equalities with their corresponding general equations.
HintsAn example of the Associative Property of Addition is,
- $(2+3)+1=2+(3+1)$
- $6=6$
An example of the Commutative Property of Addition is,
- $2+3=3+2$
- $5=5$
The associative property of multiplication and the commutative property of multiplication are the same as the addition properties but with multiplication instead of addition.
Solution1. Associative Property of Addition states that the result will be the same regardless of how the numbers are grouped.
- $(a+b)+c=a+(b+c)=b+(a+c)$
- If $a=1$, $b=2$, $c=3$,
- $(a+b)+c=(1+2)+3=3+3=6$
- $a+(b+c)=1+(2+3)=1+5=6$
- $b+(a+c)=2+(1+3)=2+4=6$
- $(ab)c=a(bc)=b(ac)$
- If $a=1$, $b=2$, $c=3$,
- $(ab)c=(1 \cdot 2)\cdot 3=2 \cdot 3= 6$
- $a(bc)=1\cdot (2\cdot 3)=1 \cdot 6= 6$
- $b(ac)=2 \cdot (1\cdot 3)=2 \cdot 3= 6$
- $a+b=b+a$
- If $a=1$ and $b=2$,
- $a+b=1+2=3$
- $b+a=2+1=3$
- $ab=ba$
- If $a=1$ and $b=2$,
- $ab=1\cdot 2=2$
- $ba=2\cdot 1=2$
-
Determine which statements are true about properties of equalities.
Hints- Associative properties- the order in which the numbers are grouped does not matter.
- Commutative properties- the order of the numbers does not matter as long as the signs remain the same.
- Equation properties- if you add/subtract a value to one side of the equation, you have to add/subtract the same value to the other side of the equation to keep them equal.
Examples:
- Associative Property of Addition: $(1+2)+3=1+(2+3)$
- Associative Property of Multiplication: $(1\cdot 2)\cdot 3=1\cdot (2\cdot 3)$
- Commutative Property of Addition: $1+2=2+1$
- Commutative Property of Multiplication: $1\cdot 2=2\cdot 1$
Addition Property of Equality: If $a=b$ and you want to add $1$, you have to add it to both sides of the equation, $a+1=b+1$
Subtraction Property of Equality: If $a=b$ and you want to subtract $1$, you have to subtract it to both sides of the equation, $a-1=b-1$.
SolutionTrue Statements
- $(2+3)+7=2+(3+7)$ is the associative property of addition.
- The addition property of equality states that if $a=b$, then $a+c=b+c$.
- $12\cdot 4=4\cdot 12$ is the commutative property of multiplication.
The commutative property of addition states that $a+b=c+a$.
- This statement is false because the commutative property of addition states that $a+b=b+a$.
- This statement is false because $7\cdot 4=4\cdot 7$ is the commutative property of multiplication.
- This statement is false because the subtraction property of equality states that if $a=b$, then $a-c=b-c$.
-
Label each calculation with the property of equality being used.
Hints- Associative properties- the order in which the numbers are grouped does not matter.
- Commutative properties- the order of the numbers does not matter as long as the signs remain the same.
Examples:
- Associative Property of Addition: $(15+16)+17=15+(16+17)$
- Associative Property of Multiplication: $(15\cdot 16)\cdot 17=15\cdot (16\cdot 17)$
Examples:
- Commutative Property of Addition: $15+16=16+15$
- Commutative Property of Multiplication: $15\cdot 16=16\cdot 15$
SolutionAssociative Property of Addition
Associative(+)
$(a+b)+c=a+(b+c)$
- $(15+26)+2=15+(26+2)$
- $(54+42)+76=54+(42+76)$
Associative(x)
$(ab)c=a(bc)$
- $(21\cdot 5)\cdot 12=21\cdot(5\cdot 12)$
Commutative(+)
$a+b=b+a$
- $99+17=17+99$
Commutative(x)
$ab=ba$
- $55\cdot 67=67\cdot 55$
- $100\cdot 68=68\cdot 100$
-
Identify which expressions equal the given values.
HintsIf we are given the expression, $5+(2+1)$, order of operations tells us to add the parentheses first:
- $5+(2+1)=5+3=8$
Notice that the order in which we add numbers does not change the result.
- $5+2=7$
- $2+5=7$
Multiplication works the same way as addition.
Order of operations tells us to multiply the parentheses first:
- $2\cdot (4\cdot 3)= 2\cdot 12=24$
- $3\cdot 2=6$
- $2\cdot 3=6$
Solution$(5+1)+6=12$ and $5+(1+6)=12$
- Notice that the sum of a group of numbers is the same regardless of how they are grouped. This is called the associative property of addition.
$4\cdot(3\cdot 1)=12$ and $(4\cdot 3)\cdot 1=12$
- Notice that the product of a group of numbers is the same regardless of how they are grouped. This is called the associative property of multiplication.
$9+8=17$ and $8+9=17$
- Notice that the sum of two numbers is the same regardless of what order they are in. This is called the commutative property of addition.
- Notice that the product of two numbers is the same regardless of what order they are in. The is called the commutative property of multiplication.
-
Decide which property of equality is being used in each step of the calculation.
HintsExample of Addition Properties:
Given: $(3+2x)+4=15$
- $(3+4)+2x=15$ Associative Property of Addition
- $2x+7=15$ Commutative Property of Addition
- $2x+9=17$ Addition Property of Equality
Example of Subtraction Property:
Given: $2x+9=17$
- $2x=8$ Subtraction Property of Equality
Example of Multiplication and Division Properties:
Given: $2x=8$
- $x\cdot 2=8$ Commutative Property of Multiplication
- $x=4$ Division Property of Equality
SolutionGiven: $(120+5d)-45=350$
1. $(120-45)+5d=350$
- Associative Property of Addition because different terms on the left side are grouped together.
- Commutative Property of Addition because the terms on the left side switch order.
- Addition Property of Equality because $10$ is added to both sides to maintain equality.
- Subtraction Property of Equality because $85$ is subtracted from both sides to maintain equality.
- Commutative Property of Multiplication because the terms on the left side switch order.
- Division Property of Equality because $5$ was divided from both sides of the equation.