Volume with Fractional Edge Lengths and Unit Cubes

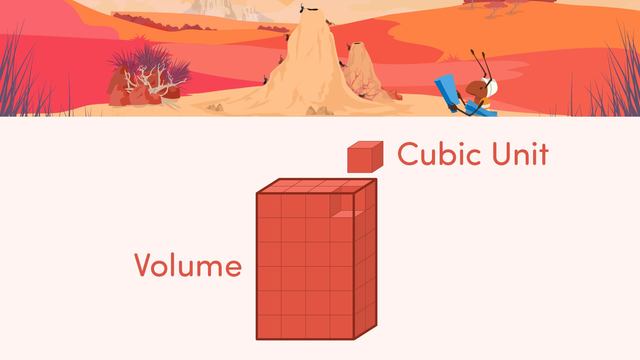
Ollie Slider is practicing tricks at his favorite skate park. The foam pit makes it easy to rehearse radical maneuvers! Oh look, it's Ollie's pet gecko, Django! Ollie built Django a ramp and pit so that he can be a pro skater too! He just needs to fill it with foam cubes. To do so, Ollie will need to calculate the volume of a rectangular prism with fractional edge lengths. How do we find the volume of a rectangular prism? Let's first look at Ollie's foam pit. It's dimensions are 3 feet by 7 feet by 8 feet. To find the volume, we need to calculate the number of unit cubes that fill the prism. Each unit cube has an edge length of 1 foot. How many cubic feet will fill this foam pit? To start we can fill the bottom layer with cubes. There are 3 rows of 7 cubes. That's 21 cubes on the bottom layer. We have 8 layers so filling the pit to the top gives us 21 times 8 which is 168 unit cubes. That give us the volume of Ollie's pit to be 168 feet cubed. Another method, is to use the formula - length, times width, times height - and see that we get the same result. So, using the formula, the volume equals 3... times 7... times 8. That gives us 168 feet cubed, just like before. Let's apply both of these methods to find the volume of Django's pit. Django's pit is smaller, and has fractional edge lengths. It's dimensions are one-half one and three-fourths and three and a half feet. First, let's calculate the volume using the formula because all we have to do is substitue the known dimensions into volume equals length, times width, times height. For Django's pit, the length is three halves the width is one and three fourths and the height is five halves. We need to convert the mixed number to an improper fraction so we can multiply. 1 and 3 fourths is the same as 4 fourths plus 3 fourths. So we can write that as 7 fourths. Therefore, the volume is three-halves times seven-fourths times five-halves. Now we multiply the numerators and denominators. This results in a volume of one hundred and five sixteenths. To rewrite as a mixed number we see that 16 goes into 105 6 times, with a remainder of nine. So, one hundred five sixteenths is equal to six and nine-sixteenths cubic feet. We can't literally fill Django's foam pit with six large unit cubes of foam and a nine-sixteenths' slice of a unit cube. Let's use the other method we know for finding volume, and fill Django's pit with smaller cubes. But what dimensions should the small cubes have? We know that the dimensions of the pit are three halves seven fourths and five halves. We need to find a fraction which will divide into each of the dimensions of the pit. Looking at the denominators we notice that the lowest common denominator is 4. So, one fourth is a fraction which divides evenly into the dimensions of the pit. Three-halves divided by one-fourth is six. Seven-fourths divided by one-fourth is seven. Five-halves divided by one-fourth is ten. So, we can use small cubes which measure one fourth foot on each edge to fill this prism. To calculate the volume using these cubes let's first figure out how many cubes fill the pit. First, we'll fill the bottom layer. There are six rows of seven small cubes in the bottom layer. That's 42 small cubes. And there are 10 layers, so the volume of the pit is 42, times 10, which is 420 small cubes and then multiply that by the total number of small cubes, 420. That means we take one-fourth times one-fourth times one-fourth and multiply it by 420. This simplifies to one-sixty-fourth times 420, which reduces to one hundred and five sixteenths. Since our problem was originally written as a mixed number we should also write our solution as a mixed number. That means six and nine-sixteenth cubic feet, just like before. Let's review how to find the volume of a rectangular prism. We can always use the formula, Volume equals length times width times height. We can also calculate the volume by filling the prism with cubes. But when the prism has fractional dimensions, we may want to measure the volume using smaller cubes. We find the dimensions for the smaller cubes by finding a fraction which will divide into each of the dimensions of the prism, like we did for Django's pit. We can give our volume in terms of smaller cubes by multiplying the number of small cubes which fill the prism by the volume of one small cube. Django's foam pit is ready to go, and so is Django. He's gonna try a wicked tail grab! Oh no! It's cool, he's a gecko, it'll grow back!