Different Types of Symmetry—Let's Practice!

Basics on the topic Different Types of Symmetry—Let's Practice!
Today we are identifying different types of symmetry with Razzi! This video contains examples to help you further practice and grow confident in this topic.
Transcript Different Types of Symmetry—Let's Practice!
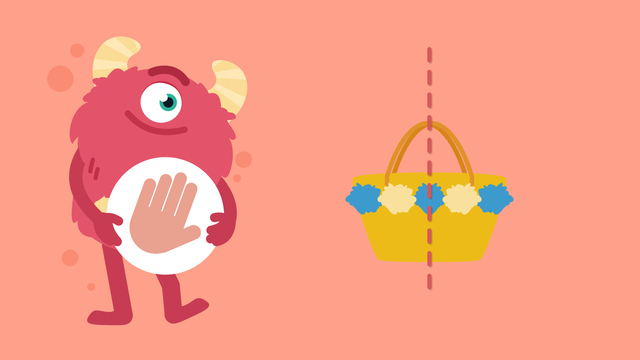
Razzi says get these items ready (...) Because today we're going to practice identifying... Different Types of Symmetry. It's time to begin! Does this wallpaper have reflexive, translational, or rotational symmetry? Pause the video to work on the problem (...) and press play when you are ready to see the solution! Since the shapes create a pattern in the same direction AND the same distance apart... it has translational symmetry. Did you also guess translational symmetry? Let's try another! Does this object have reflexive, translational, or rotational symmetry? Pause the video to work on the problem (...) and press play when you are ready to see the solution! Since one half of the object or shape reflects the other half... it has reflexive symmetry. Did you also guess reflexive symmetry? For the last problem... Which one of these pictures is an example of rotational symmetry? Pause the video to work on the problem (...) and press play when you are ready to see the solution! Since we can rotate the star around a central point HERE and it looks exactly the same... it has rotational symmetry. Razzi had so much fun practicing with you today! See you next time!
Different Types of Symmetry—Let's Practice! exercise
-
Select the correct definition.
HintsThink about the word reflexive in reflexive symmetry. What does the word reflexive sound like?
Rotational symmetry has a central point , while translational symmetry has a pattern the same distance apart.
SolutionReflexive Symmetry: one half of the object reflects the other, shown on the left.
Translational Symmetry: patterns are created in the same direction the same distance apart, shown on the right.
-
What form of symmetry is shown?
HintsThis picture can be rotated around it's central point, and still looks the same.
A star is an example of rotational symmetry.
Translational symmetry has a pattern that repeats.
SolutionThe picture of the sun has rotational symmetry.
Since the sun can be rotated around the central point and always look the same, we know this is rotational symmetry.
-
What form of symmetry do these shapes have?
HintsNone of these shapes show translational symmetry, since there are no repeating patterns.
Look at all these shapes. Do they create patterns? Can they be rotated? Does one half reflect the other?
SolutionThese shapes all show reflexive symmetry, since one half of the object reflects the other half.
-
Identify the form of symmetry shown.
HintsLook at the image now. Do you see a pattern?
This image creates a pattern in the same direction, spaced the same distance apart.
SolutionThe picture of the neighborhood shows translational symmetry, since the pattern is going in the same direction, and is spaced the same distance apart.
-
Which symmetry does the picture show?
HintsOne half of the flower reflects the other half.
The dotted line on the flower is the line of symmetry.
SolutionThe picture of the flower has reflexive symmetry, since one half of the object reflects the other half.
-
Write or type the form of symmetry shown.
HintsReflexive symmetry is where one half of the object reflects the other half.
Rotational symmetry is where an object can rotate in any direction and still have a central point of symmetry.
SolutionSince the picture of the butterfly shows one half of the object reflecting the other, this is an example of reflexive symmetry.
Since the leaves create a pattern in the same direction with the same distance apart, this is an example of translational symmetry.
Since the hexagon can be rotated around the central point and not change, this is an example of rotational symmetry.













