Counting Problems

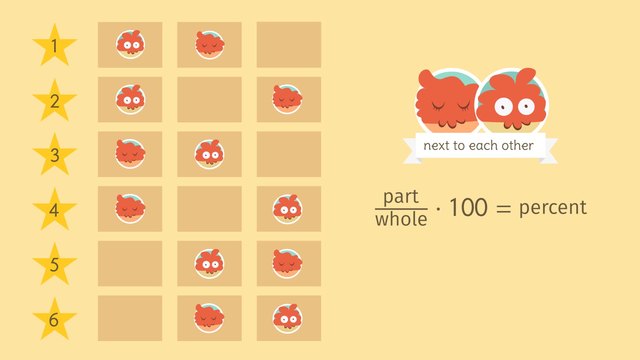
Terence has a habit of embarassing himself in front of his classmate Magnolia. This time, not only has he just shut a tentacle in his locker door, but in the shock of the moment, he forgot his locker combination! Let's see if we can save Terence some embarrassment by helping him out of this Counting Problem. Terence's locker combination consists of three different numbers: 12, 49, and 37. And Terence knows that the combination ends with the number 49. If each number is used only once, what percent of all possible locker combinations involving 12, 49, and 37, end with the number 49? To answer that question, we need to find out two things: Our whole, our part, then, we'll be able to calculate a percent. Let's start by determining the whole. What do you think represents our whole, or total, in this problem? Since we know the numbers 12, 49, and 37 are each used once, our whole will be all the possible locker combinations containing only those numbers. Given this list of all possible outcomes, we can see that there are 1, 2, 3, 4, 5, 6 possible combinations which use our 3 numbers. These 6 combinations represent the whole. If we are looking for the percent of combinations that end with the number 49, what do you think is the "part" in this problem? Take a look at the table and count how many combinations end in the number 49. Looks like these two fit the bill, so 2 will be our part. Two combinations out of 6 equals two-sixths, or one-third. That means only about 33.3 percent of all possible combinations end with the number 49. Magnolia doesn't seem fazed by the whole tentacle incident, so she and Terence head off to their next class. But it looks like there are just three open seats left, which means there are only a few possible seating arrangements for them to choose from. In what percent of these do Terence and Magnolia end up sitting next to each other? Just like before, we need to identify the part and the whole in order to calculate a percent. So, what do you think represents our "whole" in this scenario? Looking at these different seating arangements, we can see there are 6 possible outcomes total, so that will represent our whole. Now, what should the "part" be in this problem? We want to know the percent of seating arrangements that have Terence and Magnolia next to each other. So the part is the number of outcomes that match this description. Looking in our chart, we can see that Terence is next to Magnolia in 1, 2, 3, 4 out of 6 possible options. Now that we know the part and the whole, we can calculate the percent. 4 out of 6 possible outcomes is four-sixths, or two-thirds. Rounding off our decimal, that means in about 66.7 percent of all seating arrangements, Terence and Magnolia end up next to each other. So, while Terence snags a seat, let's review counting problems. When dealing with counting problems, remember that you're really just working with parts, wholes, and percents. Given a list of all possible outcomes, count the total number of outcomes to find the whole. That number goes in your denominator. Then, figure out how many outcomes fit the criteria you're looking for. This number is the part, so it goes in the numerator. Finally, you can calculate the percent. Back in class with Terence and Magnolia, I don't think that's the outcome that Terence was hoping for. But maybe that new kid is nice?