Adding, Subtracting, Multiplying, and Dividing Rational Expressions

Basics on the topic Adding, Subtracting, Multiplying, and Dividing Rational Expressions
Operations with rational expressions are essentially the same as operations with fractions. One is just dealing with polynomials instead of numbers in the numerator and denominator of the expressions. But the rules for adding, subtracting, multiplying, and dividing, however, are very much the same.
For addition and subtraction of rational expressions, the least common denominator, or LCD, of the expressions must be found. To find the LCD, we factor the denominators to find the unique factors which appear in all denominators and multiply these together to get the LCD. The equivalent numerators are derived by multiplying all the terms of the expressions with the factors (as a fraction equivalent to 1) not found in each corresponding denominator. All the numerators can then be added or subtracted since they are now similar, since their corresponding expressions all have the same denominator now. Simplifying the rational expression in the answer, when applicable, is also recommended.
When multiplying, we can just as easily multiply the numerators of the rational expressions and the denominators as well. Factoring also helps to eliminate common factors in the numerator and denominator so that the answers we initially get will already be the final answer without the need to simplify.
Division, being the inverse of multiplication, is done by flipping the numerator and denominator of the second expression. The expressions are then solved like a regular multiplication operation.
Rational expressions may look a bit daunting to solve, but operations on rational expressions are really quite easy when you know all the techniques.
Rewrite Rational Expressions.
CCSS.MATH.CONTENT.HSA.APR.D.7
Transcript Adding, Subtracting, Multiplying, and Dividing Rational Expressions
Chris and two of his best friends formed a group to build a drone for their school’s science fair project. There’s just one catch: this year for the science fair, all the students must use rational expressions and four different operations in order to create their project. The students’ entries will be judged by how well adding, subtracting, multiplying and dividing rational expressions is incorporated in their projects. Unfortunately, Chris and his friends were too busy goofing around and having fun. As night falls, and Chris’s friends all go home, Chris realizes that he must complete the project by himself.
His dad, being an engineer, taught him to approach problems one step at a time. Thinking that this is pretty good advice, Chris decides to take a closer look at his project. There are so many wires to connect, what’s Chris to do? He must perform each of the operations to the pairs of rational expressions on the control pad of the drone and connect the wires to the correct answers in order to get the drone working.
Finding the least common denominator
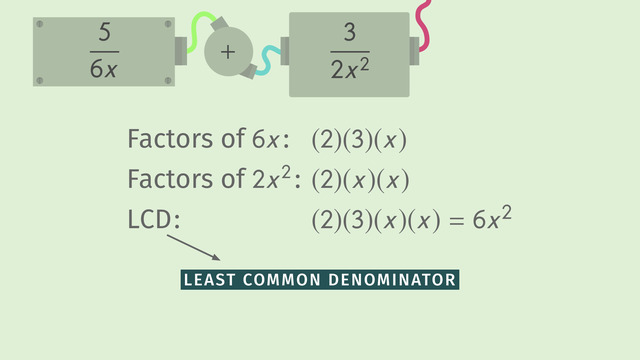
He investigates the label for the first set of wires and quickly realizes what he must do. He has to add the two rational expressions given to him! Chris knows the first thing he needs to do when adding fractions is to find a least common denominator for each of the fractions. To do this, Chris first lists the factors of the two denominators he's been given. The least common denominator is 2 times 3 times 'x' times 'x', or 6x squared.
Simplyfing fractions
Next, he multiplies each fraction by a factor that's not in the denominator. He multiplies 5 over 6x by 'x' over 'x' and multiplies 3 over 2x squared by 3 over 3. Now that the two fractions have the same denominator, Chris can safely add the two numerators and then simplify, if needed.
Since 5, 6 and 9 don't share any common factors, Chris can't simplify the fraction any further. Awesome. One down, three to go. The next set of wires requires Chris to subtract two rational expressions. Luckily, the process for subtraction is the same as for addition.First, Chris must make sure the denominators are the same, so he lists the factors of 4x and 3x plus 1. This doesn't help much, so to find the least common denominator for this expression, Chris multiplies the two denominators together. Chris has to multiply the first fraction by 3x plus 1 over 3x plus 1. The second rational expression has to be multiplied by 4x over 4x. Using the distributive property, Chris multiplies the terms in each of the numerators.
After subtracting the numerators, Chris remembers that he's supposed to write expressions in decreasing exponential order. Two down, two to go. Chris is making excellent progress!
Canceling out
The next set of wires requires Chris to multiply. Thankfully, he doesn't have to worry about LCDs anymore. All he has to do now is multiply the two numerators and the two denominators. Chris lists the factors of the numerator and the denominator separately. The next part is Chris's favorite. Time to cancel out similar factors! If you can find a term in both the numerator and the denominator, you can cancel them out!
There's one 2 and two 'x's that are in both the numerator and denominator, so Chris cancels out these terms, giving him 5x over 12. That was quick. Uh-oh, the sun's starting to peek over the horizon! It's almost morning! Chris had better get a move on if he wants to finish the wiring on time.
Dividing rational expressions
The last wire has two choices. Should he just try them both and see which one works? Chris decides to just work the problem. He can't afford to mess up his project now! Just like when dividing regular fractions, dividing rational expressions is no different. Chris just changes it into a multiplication problem and flips the second rational expression. Since the numerator and denominator don't share any common terms, Chris can just multiply everything in the numerator together and then FOIL the denominator and he's done!
Just as the sun rises, Chris quickly hooks up the last set of wires. Whew! Done just in time! As Chris enters the science fair area, however. It seems building a drone was a popular choice for this year’s science fair. The winner’s even amazed!
Adding, Subtracting, Multiplying, and Dividing Rational Expressions exercise
-
Explain how to add or subtract rational expressions.
HintsWe have that $\frac{5}{6x}+\frac3{2x^2}=\frac{5x+9}{6x^2}$.
Let's have a look at another example:
$\frac32-\frac53$
You can only combine (add or subtract) fraction with the same denominator. So in order to make these two fractions have the same denominator, expand the first fraction by $3$ and the second by $2$:
$\frac32-\frac53=\frac{(3)(3)}{(2)(3)}-\frac{(5)(2)}{(3)(2)}=\frac{9}{6}-\frac{10}{6}=-\frac16$
Here is an example for factoring a given term.
SolutionTo add or subtract rational expressions we first have to find the LCD, or
the Least Common Denominator.
How can we find it?
- First we factor the denominators of the given fractions.
- Next we find the LCD by taking all given factors of the denominators. But only take factors which appear in both denominators only once.
$\frac{5}{6x}+\frac3{2x^2}$
- $6x=(2)(3)(x)$ and $2x^2=(2)(x)(x)$, so the factors $2$ as well as $x$ appear in both denominators. So we take each of these only once.
- The corresponding LCD is given by $(2)(3)(x)(x)=6x^2$.
- We expand the first fraction by $x$ to get $\frac{5}{6x}=\frac{(5)(x)}{(6x)(x)}=\frac{5x}{6x^2}$
- We expand the second one by $3$, which results in $\frac{3}{2x^2}=\frac{(3)(3)}{(2x^2)(3)}=\frac{9}{6x^2}$
$\frac{5}{6x}+\frac3{2x^2}=\frac{5x}{6x^2}+\frac{9}{6x^2}=\frac{5x+9}{6x^2}$.
-
Multiply and divide the following rational expressions.
HintsHere is an example of multiplying two fractions:
To multiply two binomials use the FOIL method:
$(x+1)(x+2)=x\times x+x\times 2+1\times x+1\times 2=x^2+3x+2$.
Here is an example of the dividing two fractions:
$\begin{array}{rclll} \frac{2}{3}\div\frac{5}{4}&=&~\frac23\times \frac45&|&\text{multiply with the flipped second fraction}\\ &=&~\frac{(2)(4)}{(3)(5)}\\ &=&~\frac{8}{15} \end{array}$
SolutionMultiplying or dividing two fractions is a little bit easier than adding or subtracting. You don't have to determine the least common denominator.
In order to multiply, you only have to multiply the two numerators as well as the denominators:
$\frac{5}{8x^2}\times\frac{2x^3}{3}=\frac{(5)(2x^3)}{(8x^2)(3)}$
Next, list the factors of the numerator as well as of the denominator:
$\frac{(5)(2x^3)}{(8x^2)(3)}=\frac{(5)(2)(x)(x)(x)}{(2)(2)(2)(x)(x)(3)}$,
and cancel out those which appear in both:
$\frac{(5)(\not 2)(\not x)(\not x)(x)}{(2)(2)(\not 2)(\not x)(\not x)(3)}=\frac{(5)(x)}{(2)(2)(3)}=\frac{5x}{12}$
For dividing two fractions, you first have to flip the second one and then you can multiply in the same way like before:
$\frac{4x^3}{x+1}\div\frac{x+2}{x}=\frac{4x^3}{x+1}\times\frac{x}{x+2}$
Multiply the numerators as well as the denominators:
$\frac{4x^3}{x+1}\times\frac{x}{x+2}=\frac{(4x^3)(x)}{(x+1)(x+2)}$
Because we can't simplify this fraction by cancelling out, we still have to multiply the binomials in the denominator:
$\frac{(4x^3)(x)}{(x+1)(x+2)}=\frac{4x^4}{x^2+3x+2}$
-
Find the expressions that can be simplified.
HintsLet's have a look at the following example $\frac46$.
The numerator contains the factor $2$ as well as the denominator. So you can cancel it out.
Here you see how to cancel a common factor out.
In general, factor both the numerator as well as the denominator. Check factors in common and cancel those common factors out.
SolutionHow can we decide if a given fraction could be simplified?
First, factor the numerator as well as the denominator. If both have one or more factors in common, then those can be cancelled out. This is how you simplify an expression. For
$\frac{2x^2}{3x}=\frac{(2)(x)(x)}{(3)(x)}$
we can find the factor $x$ in the numerator as well as in the denominator.
On the other hand, $\frac{2x^2}{3}=\frac{(2)(x)(x)}{3}$ has no factors in common and thus can't be simplified.
There could also be more than one variable:
$\frac{6x}{4a}=\frac{(2)(3)(x)}{(2)(2)(a)}$
Here we have the common factor $2$, which could be cancelled out.
Remember that we can only cancel out factors.
For $\frac{6x+1}{4a}=\frac{6x+1}{(2)(2)(a)}$ there is no common factor.
Lastly, let's have a look at an example where more than one factor which could be cancelled out:
$\begin{array}{rclll} \frac{12x}{6x^2}&=&~\frac{(2)(2)(3)(x)}{(2)(3)(x)(x)}&|&\text{factoring}\\ &=&~\frac{(\not 2)(2)(\not 3)(\not x)}{(\not 2)(\not 3)(\not x)(x)}&|&\text{cancelling out}\\ &=&~\frac2x \end{array}$
-
Determine the following expression.
HintsTo multiply two fractions, multiply their numerators as well as their denominators.
Here you see how to divide fractions. Just multiply with the flipped fraction.
Simplify the fractions if it's possible. That means cancel common factors in the numerator and the denominator out.
You could add or subtract from left to the right according to PEMDAS:
Start with $\frac{5}{12x}+\frac{x}{3}$. First determine the LCD:
- $12x=(2)(2)(3)(x)$
- $3=(3)$
$\frac{5}{12x}+\frac{x}{3}=\frac{5}{12x}+\frac{x(4x)}{(3)(4x)}=\frac{5}{12x}+\frac{4x^2}{12x}=\frac{4x^2+5}{12x}$.
SolutionThis term looks a little bit more complicated.
First, keep PEMDAS in mind: let's sketch the calculation:
- $\frac{4}{x^3} \times \frac{x}{18}$
- Divide the product by $\frac{2}{3x^3}$
- From left to the right do the addition (or subtraction).
$\begin{array}{rclll} \frac{4}{x^3} \times \frac{x}{18}&=&~\frac{(4)(x)}{(x^3)(18)}&|&\text{factoring}\\ &=&~\frac{(2)(2)(x)}{(2)(3)(3)(x)(x)(x)}&|&\text{cancelling out}\\ &=&~\frac{2}{9x^2} \end{array}$
Next, divide the resulting fraction by $\frac{2}{3x^3}$. For this we multiply the resulting fraction with the flip of the fraction we are dividing by:
$\begin{array}{rclll} \frac{2}{9x^2}\div \frac{2}{3x^3}&=&~\frac{2}{9x^2}\times \frac{3x^3}{2}&|&\text{multiply with the flipped fraction}\\ &=&~\frac{(2)(3x^3)}{(9x^2)(2)}&|&\text{factoring}\\ &=&~\frac{(2)(3)(x)(x)(x)}{(2)(3)(3)(x)(x)}&|&\text{cancelling out}\\ &=&~\frac{x}{3} \end{array}$
Now we can add or subtract them from left to the right:
$\frac{5}{12x}+\frac{x}{3}-\frac{x}{3}=\frac{5}{12x}$.
-
Explain the meaning of LCD.
HintsTo determine the LCD first factor, as you can see pictured.
The LCD is $(2)(2)(2)(3)=24$.
To check it, you can divide $24$ by $8$ as well as by $12$.
You can only add or subtract fractions with common denominators.
To multiply two fractions, just multiply the numerators as well as the denominators.
The result of the addition above is given by
$\begin{array}{rcl} \frac{5}{12}+\frac{3}{8}&=&~\frac{(5)(2)}{(12)(2)}+\frac{(3)(3)}{(8)(3)}\\ &=&~\frac{10}{24}+\frac{9}{24}\\ &=&~\frac{19}{24} \end{array}$
SolutionTo add or subtract two fractions we first have to determine the LCD, or least common denominator. How can we determine it?
Let's have a look at the following example: $\frac{5}{6x}+\frac3{2x^2}$.
- Factor both denominators $6x=(2)(3)(x)$ and $2x^2=(2)(x)(x)$ The factors $2$ as well as $x$ appear in both denominators. So we take them only once.
- The LCD is given by $(2)(3)(x)(x)=6x^2$.
-
Calculate the given expressions.
HintsFor adding or subtracting fractions you have to find the LCD of their denominators.
Let's find the LCD of the denominators of the fractions in the expression $\frac{2}{3x}+\frac{4}{6}$:
- $3x=(3)(x)$
- $6=(2)(3)$
Expand each fraction so that we have the LCD as the common denominator.
To multiply two fractions, multiply the numerators as well as the denominators.
To divide two fractions, $\frac ab\div \frac cd$, multiply the first one with the flip of the second one $\frac ab\times\frac dc$.
SolutionHere we practice each operation once.
For the addition of fractions:
$\frac{4x^2}{9}+\frac{2}{3x}$.
For this we first determine the LCD as follows:
- $9=(3)(3)$
- $3x=(3)(x)$
$\begin{array}{rclll} \frac{4x^2}{9}+\frac{2}{3x}&=&~\frac{(4x^2)(x)}{(9)(x)}+\frac{(2)(3)}{(3x)(3)}\\ &=&~\frac{4x^3}{9x}+\frac{6}{9x}&|&\text{add the numerators}\\ &=&~\frac{4x^3+6}{9x} \end{array}$
For subtraction we proceed in a similar manner:
$\begin{array}{rclll} \frac{9}{4x^2}-\frac{4}{3x}&=&~\frac{(9)(3)}{(4x^2)(3)}-\frac{(4)(4x)}{(3x)(4x)}&|&\text{LCD: } 12x^2\\ &=&~\frac{27}{4x^2}-\frac{16x}{12x^2}&|&\text{subtract the numerators}\\ &=&~\frac{-16x+27}{12x^2} \end{array}$
Keep in mind that terms get written in descending order of the exponents.
For the multiplication of two fractions, multiply the numerators as well as the denominators.
$\begin{array}{rclll} \frac{9}{4x^2}\times\frac{4}{3x}&=&~\frac{(9)(4)}{(4x^2)(3x)}&|&\text{factoring }\\ &=&~\frac{(2)(2)(3)(3)}{(2)(2)(3)(x)(x)(x)}&|&\text{cancelling out}\\ &=&~\frac{3}{x^3} \end{array}$
Last but not least, we look at the division of two fractions. For this multiply by the flip of the second one.
$\begin{array}{rclll} \frac{15}{4x+4}\div\frac{21}{5x+5}&=&~\frac{15}{4x+4}\times\frac{5x+5}{21}\\ &=&~\frac{(15)(5x+5)}{(4x+4)(21)}&|&\text{factoring}\\ &=&~\frac{(3)(5)(5)(x+1)}{(2)(2)(3)(7)(x+1)}&|&\text{cancelling out}\\ &=&~\frac{(5)(5)}{(2)(2)(7)}=\frac{25}{28} \end{array}$