The Converse of the Pythagorean Theorem
- The Converse of the Pythagorean Theorem
- The Converse of the Pythagorean Theorem – Explanation
- The Converse of the Pythagorean Theorem – Examples
- The Converse of the Pythagorean Theorem – Practice
- The Converse of the Pythagorean Theorem – Summary
- The Converse of the Pythagorean Theorem – Frequently Asked Questions

Basics on the topic The Converse of the Pythagorean Theorem
The Converse of the Pythagorean Theorem
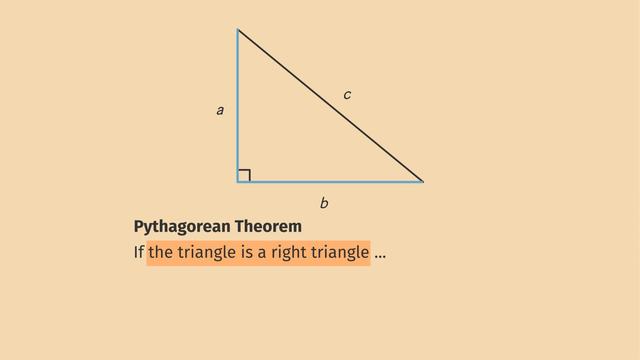
The converse of the Pythagorean Theorem is an important idea in geometry. It helps us figure out if a triangle has a right angle. If adding the squares of the two shorter sides equals the square of the longest side, then the triangle is a right triangle. Applying the Pythagorean Theorem is very useful for solving math problems and is also used in many real-life situations where right angles are needed.
The Converse of the Pythagorean Theorem states that for any triangle with sides of lengths a, b, and c (where c is the longest side), if a2 + b2 = c2 holds true, then the triangle is a right triangle with the right angle opposite side c.
The Converse of the Pythagorean Theorem – Explanation
The converse of the Pythagorean Theorem helps confirm the nature of a triangle by relating the squares of its side lengths. This property is especially useful in fields like construction, where confirming right angles is crucial for the integrity of structures.
| Step | Description | Detail |
|---|---|---|
| 1 | Identify the sides | Determine which side would be the hypotenuse if the triangle were right-angled. This is always the longest side, $c$. |
| 2 | Apply the theorem | Square the lengths of the shorter sides ($a$ and $b$) and sum them up. |
| 3 | Compare the sum to $c^{2}$ | If $a^{2} + b^{2}$ equals $c^{2}$, the triangle is right. |
The Converse of the Pythagorean Theorem – Examples
Let’s practice some together.
Example 1: Triangle with sides 6 units, 8 unit, and 10 units
- Identify the sides: The longest side, which would be the hypotenuse in a right triangle, is $c = 10$ units.
- Apply the theorem: Calculate and sum the squares of the other two sides: $a^{2} = 6^{2} = 36$ and $b^{2} = 8^{2} = 64$. The sum is $a^{2} + b^{2} = 36 + 64 = 100$.
- Compare the sum to $c^{2}$: Since $c^{2} = 10^{2} = 100$ and $a^{2} + b^{2} = c^{2}$, by the converse of the Pythagorean Theorem, the triangle is a right triangle.
Example 2: Triangle with sides 5 units, 12 units, and 13 units
- Identify the sides: The longest side, which would be the hypotenuse in a right triangle, is $c = 13$ units.
- Apply the theorem: Calculate and sum the squares of the other two sides: $a^{2} = 5^{2} = 25$ and $b^{2} = 12^{2} = 144$. The sum is $a^{2} + b^{2} = 25 + 144 = 169$.
- Compare the sum to $c^{2}$: Since $c^{2} = 13^{2} = 169$ and $a^{2} + b^{2} = c^{2}$, by the converse of the Pythagorean Theorem, the triangle is a right triangle.
Let’s apply the converse of the Pythagorean Theorem to determine if a triangle with sides of 5 units, 12 units, and 13 units is a right triangle.
Is the triangle with sides measuring 9 units, 12 units, and the longest side (hypotenuse) measuring 15 units a right triangle? Let’s apply the converse of the Pythagorean Theorem!
Can a triangle with side lengths of 7 units, 24 units, and a hypotenuse of 25 units be classified as a right triangle? Use the Pythagorean Theorem to find out.
The Converse of the Pythagorean Theorem – Practice
The Converse of the Pythagorean Theorem – Summary
Key Learnings from this Text:
- The converse of the Pythagorean Theorem allows us to verify whether a triangle is a right triangle.
- By verifying that $a^{2} + b^{2} = c^{2}$, where $c$ is the longest side, we can conclusively determine the triangle's right-angle nature.
The Converse of the Pythagorean Theorem – Frequently Asked Questions
Transcript The Converse of the Pythagorean Theorem
Rana is studying interior dungeon design at Fantasy U. With her dragon, Rekhi, by her side, Rana is building shelving brackets for her new dorm room. To be stable, the brackets must form RIGHT triangles. But Rekhi melted Rana's protractor, so she can't measure the angles. Nevertheless, Rana can work backwards and use the CONVERSE of the Pythagorean Theorem to determine if her brackets are RIGHT. Remember that the Pythagorean Theorem tells us that, IF the triangle is a right triangle and we call the legs 'a' and 'b' and the hypotenuse 'c' THEN 'a' squared plus 'b' squared equals 'c' squared. The CONVERSE of the Pythagorean Theorem reverses the order. IF 'a' squared plus 'b' squared equals 'c' squared THEN the triangle is a right triangle. In order to use the converse statement with confidence, we first need to PROVE that it's true. We'll use a technique called INDIRECT PROOF to do that. For any proof we always start with writing our statement we want to prove. To prove the converse of the pythagorean theorem means we're proving that if a triangle follows 'a' squared plus 'b' squared equals 'c' squared, then it is a right triangle. Next since we're doing an indirect proof, we assume the opposite of the statement we want to prove. Here let's ASSUME that our triangle is NOT a right triangle. We'll then use logic to show that this assumption leads to a CONTRADICTION. That contradiction will mean that our assumption was false. Therefore, the statement we wanted to prove must be true. We already have our statement that we want to prove: if a triangle follows 'a' squared plus 'b' squared equals 'c' squared, then it's a right triangle. We already know that we are going to assume that our triangle is NOT a right triangle. So what type of triangle could it be? It could be an ACUTE triangle or an OBTUSE triangle. So, for our proof let's draw an acute triangle... and an obtuse triangle. Let's label the vertices and the sides with 'A' 'B' and 'C' for both triangles. In the acute triangle, construct a perpendicular line segment to CB that is the same length as AC and call the new point 'D.' Therefore, this side length is also 'b'. We can construct the same perpendicular segment in the obtuse triangle as well. Now, we can complete our RIGHT triangles by connecting points 'D' and "B' in the acute triangle as well as in the obtuse triangle. Because these are right triangles the Pythagorean Theorem applies, so in our triangle 'a' squared plus 'b' squared equals 'DB' squared. But remember, we already know that 'a' squared plus 'b' squared equals 'c' squared. This means that we can call 'DB' 'c' in this triangle and in the other triangle. Now connect segment DA in both triangles, and recall that an isosceles triangle is a triangle that has two sides of equal length. Therefore focusing on the left diagram, we can see that we have TWO isosceles triangles here and here, right? Since ACD is an isosceles triangle, THESE two base angles are congruent. This means the measure of angle 'C', 'D', 'A' equals the measure of angle 'C', 'A', 'D'. And since triangle ABD is isosceles, THESE two base angles are congruent. This means the measure of angle 'B', 'D', 'A' equals the measure of angle 'B', 'A', 'D'. However, from the diagram, we can definitely see that angle C,D, A is GREATER than angle B,D,A. And because of THESE equations we can use the substitution property of equality, that means the measure of angle 'C', 'A', 'D' has to be greater than that of angle 'B', 'A', 'D'. But look back at the diagram: angle 'C', 'A', 'D' canNOT be greater than angle 'B', 'A', 'D', it's a CONTRADICTION! In fact, all those base angles didn't look congruent anyhow, but we had to prove they weren't congruent. Now let's look at the second diagram and using the same logic we get a similar situation because our final statement would say that angle 'C', 'D', 'A' is greater than angle 'B', 'D', 'A'. That's definitely a contradiction! Our ASSUMPTION, that our triangle WASN'T a right triangle, led to an overall CONTRADICTION, which was step four of our indirect proof. The only way for our triangle to possibly work is when it is a right triangle. Therefore, the CONVERSE of the Pythagorean Theorem is TRUE. IF 'a' squared plus 'b' squared equals 'c' squared, THEN the triangle is a right triangle. So, let's see if Rana's bracket is RIGHT. Here 'a' is 9 'b' is 12... and 'c' is 15. 9 squared is 81, 12 squared is 144, and 15 squared is 225. 81 plus 144 is 225, so 'a' squared plus 'b' squared DOES EQUAL 'c' squared. By the converse of the Pythagorean Theorem, that means the triangle is a right triangle. Rana can confidently construct a stable shelf using this bracket! Let's review! We proved the converse of the Pythagorean Theorem... using an INDIRECT PROOF. We started with the statement we wanted to prove. We assumed the converse was FALSE, and then used logic to lead to a CONTRADICTION. That meant the converse of the Pythagorean Theorem was actually TRUE. Let's see how Rana's shelf turned out! Best not to wake a sleeping dragon. That might lead to a serious contradiction!
The Converse of the Pythagorean Theorem exercise
-
Find the square of the numbers.
HintsWhen we square a number we multiply it by itself. A skill we need when we are using the Pythagorean Theorem. For example, $4^2 = 4\times4 = 16$
When we square a decimal with one decimal place the answer will have two decimal places. For example, $0.1^2 = 0.1\times0.1 = 0.01$.
Solution- $3^2 = 3\times3 = 9$. We can see this above as the area of a square with sides $3$.
- $0.5^2 = 0.5\times0.5 = 0.25$
- $5^2 = 5\times5 = 25$
- $0.2^2 = 0.2\times0.2 = 0.04$
-
Understanding the converse of the Pythagorean theorem.
HintsWe use the Pythagorean Theorem to find out if the triangle is right angled.
If, $a^2 + b^2$ is the same value as $c^2$ then the triangle is right angled.
Substitute the values into the theorem and square them.
For example, to check this triangle we use:
- $a^2 + b^2 = c^2$
- $2^2 + 3^2 = 4^2$
- $4 + 9 = 16$
- This is not correct as $4 + 9 = 13$ not $16$ therefore, the triangle is not right angled.
Solution- The Pythagorean Theorem states that $a^2 + b^2 = c^2$
- Using this triangle $7^2 + 40^2 = 42^2$
- We square all the numbers so, $49 + 1600 = 1764$
- Therefore the triangle is not right angled!
-
Use the converse of the Pythagorean Theorem.
HintsFor a triangle to be right the Pythagorean Theorem should be true. That is $a^2 + b^2 = c^2$, where $c$ is the longest side, the hypotenuse.
For example, if $a = 9$, $b = 12$ and $c = 15$ we can use the Pythagorean theorem to check if the triangle is right.
- $a^2 + b^2 = c^2$
- $9^2 + 12^2 = 15^2$
- $81 + 144 = 225$
- As $81 + 144$ does $= 225$, the triangle is right.
There are $2$ correct answers.
SolutionRight triangles are:
- $a = 3, b = 4, c = 5$ as $3^2 + 4^2 = 5^2$ as $9 + 16 = 25$ Correct
- $a = 6, b = 8, c = 10$ as $6^2 + 8^2 = 10^2$ as $36 + 64 = 100$ Correct.
- $a = 4, b = 5, c = 6$ as $4^2 + 5^2 = 6^2$ as $16 + 25 = 36$ Not correct.
- $a = 8, b = 10, c = 12$ as $8^2 + 10^2 = 12^2$ as $64 + 100 = 144$ Not correct.
-
Use the Pythaogrean theorem to prove which triangle has a right angle.
HintsFor a triangle to be right, the Pythagorean Theorem should be true. That is $a^2 + b^2 = c^2$, where $c$ is the longest side, the hypotenuse.
For example, if $a = 1$, $b = 2$ and $c = 3$ we can use the Pythagorean theorem to check if the triangle is right.
- $a^2 + b^2 = c^2$
- $1^2 + 2^2 = 3^2$
- $1 + 4 = 9$
- As $1 + 4$ does not $= 9$, the triangle is not right.
SolutionThe right triangle is:
- $a = 1.5, b = 2, c = 2.5$
- $1.5^2 + 2^2 = 2.5^2$
- $2.25 + 4 = 6.25$
_______________________________________________________
The others are not equal so are not right triangles.
For example,
- $a = 2, b = 4, c = 6$
- $2^2 + 4^2 = 6^2$
- $4 + 16 = 36$. This is not correct, so is not a right triangle.
-
Identifying the sides of a right triangle.
HintsPythagorean Theorem states that the squares off the two shorter sides added together are equal to the square off the hypotenuse.
Pythagorean Theorem is $a^2 + b^2 = c^2$, where $c$ is the hypotenuse.
The hypotenuse is the side opposite the right angle, and is the longest side in a right triangle.
As an example, we can see here that,
- $3^2 + 4^2 = 5^2$
- $3^2 = 9$
- $4^2 = 16$
- $5^2 = 25$
- $9 + 16 = 25$
SolutionThe Pythagorean Theorem is $a^2 + b^2 = c^2$ where $c$ is the hypotenuse (the longest side, opposite the right angle).
-
Indirect proof
HintsWhen completing an indirect proof of a right triangle we firstly write the statement and assume the triangle is not a right triangle.
We use the triangle to try to prove it is NOT right. That means it is not equal to $a^2 + b^2 = c^2$.
If we find the assumption is not correct, we can say it is a right triangle.
SolutionAn indirect proof is when we state the theorem, then try to prove otherwise. If we cannot prove otherwise then we can indirectly say the theorem is correct.










