A Proof of the Pythagorean Theorem
Basics on the topic A Proof of the Pythagorean Theorem
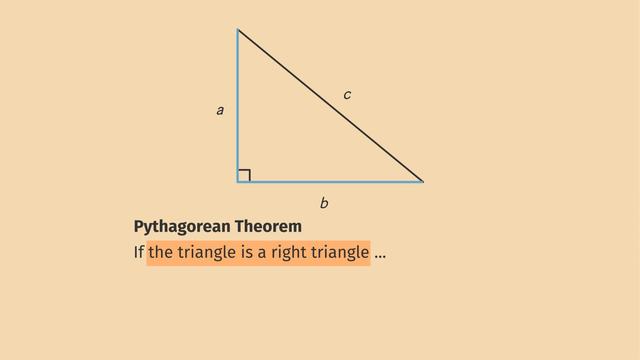
Proof of the Pythagorean Theorem
The Pythagorean Theorem is a fundamental principle in geometry, crucial for various applications in fields like architecture, engineering, and everyday problem-solving. This theorem helps in determining the length of one side of a right triangle when the lengths of the other two sides are known.
Pythagorean Theorem states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. This relationship is expressed as $a^{2} + b^{2} = c^{2}$, where $c$ is the hypotenuse and $a$ and $b$ are the other two sides.
Illustration request:
Understanding the Pythagorean Theorem
The Pythagorean Theorem is not just a mathematical rule but a bridge to understanding spatial relationships and geometry. It provides a clear method for calculating the distance between points in a plane, an essential technique in various scientific and practical applications.
Proving the Pythagorean Theorem
The beauty of the Pythagorean Theorem lies in its proof, which combines simple geometry with algebraic manipulation. Here’s how it’s typically proven:
Sure! Here's the table with an additional column for images:
| Step | Description | Example | Image |
|---|---|---|---|
| 1. | Construct a right triangle | Draw a triangle with sides of lengths 3, 4, and 5 | ILLU REQUEST: |
| 2. | Draw squares on each side | Create squares on each side of the triangle | ILLU REQUEST: |
| 3. | Calculate the area of each square | Find the area of each square using side lengths | ILLU REQUEST: |
| 4. | Show that the areas add up | Prove that the sum of the areas equals the area of the square on the hypotenuse | ILLU REQUEST: $9+16=25$ |
Proof of the Pythagorean Theorem – Practice
Let’s work through the following problem together to apply the Pythagorean Theorem:
Proof of the Pythagorean Theorem – Summary
Key Learnings from this Text:
The Pythagorean Theorem describes a fundamental relationship in right triangles.
It is expressed as $a^{2} + b^{2} = c^{2}$, where $c$ is the hypotenuse.
The theorem is not only theoretical but also practical, used in various real-world applications.
Proof of the Pythagorean Theorem – Frequently Asked Questions
Transcript A Proof of the Pythagorean Theorem
Maurice is trying to do his homework on applying the Pythagorean Theorem while watching his little sister, Sina when suddenly Eureka! The arrangement of Sina's blocks gives Maurice an idea for a proof of the Pythagorean Theorem! If we prove that the Pythagorean Theorem is true for a right triangle with variable side lengths, that will mean it will be true for right triangles of all side lengths. Remember, the Pythagorean Theorem tells us that, given a right triangle, the sum of the squares of the legs equals the square of the hypotenuse. We write this algebraically as 'a' squared plus 'b' squared equals 'c' squared. This is the theorem that we want to prove using logical statements and properties we already know. Once we prove the Pythagorean Theorem we can use it to find a missing side length. Notice that the blocks, Sina is playing with, show a large square that is actually made up of 4 right triangles and a smaller square. Each side of the large square is divided into lengths of 'a' and 'b'. To prove that the Pythagorean Theorem is true, we will calculate the area of the large square using its side lengths and set it equal to the area of the shapes it is composed of. Recall, the area of a square is just its side length squared. What is the side length of the large square? It's 'a' plus 'b'. That means the area of the large square is the quantity 'a' plus 'b' squared. Remember, squaring means multiplying by itself, so that's just 'a' plus 'b' times 'a' plus 'b'. Notice that we are multiplying two binomials. Therefore we need to distribute, which means to multiply each term in the first expression by the terms in the second. That gives us 'a' squared plus 'ab' plus 'ba' plus 'b' squared. We can simplify by combining like terms. That is 'ab' plus 'ba' is '2ab'. So, the total area of the large square is 'a' squared plus '2ab' plus 'b' squared. We can find the area of the large square by also summing up the areas of the triangles and the small square. Now, let's find the area of the triangles. Are these triangles congruent? Placing them on top of each other shows us that the triangles are in fact the same. So, we can just start by finding the area of one triangle to find the area of them all. What is the formula for the area of a triangle, again? Recall, area of a triangle is one-half the base times the height. We can substitute 'a' for the base and 'b' for the height resulting in one-half 'ab.' This is just the area for one triangle so, the total area of all four triangles is 4 times one-half 'ab'. Simplifying shows us the area of all the triangles can be expressed as '2ab'. Now let's look at the smaller square. Actually, how do we know that this is truly a square? Remember to be a square the shape must have four equal sides and four right angles. Because the four triangles are congruent, these four segments are congruent. We'll call the length of each side, 'c'. So, it has four equal sides but what about the right angles? In order to determine the value of these angles let's recall the angle sum theorem that states, the sum of the measures of the interior angles of a triangle is 180 degrees. Since we already know the triangle has one angle measure of 90 degrees it means these two acute angles... must sum to 90 degrees. Because all the triangles are congruent it means their corresponding angles are also congruent. Therefore, these two angles also sum to 90 degrees. And since this is a straight angle, measuring 180 degrees that means this angle of the inside shape must be 90 degrees. This inside shape has 4 right angles, meaning it is in fact a square. Since it is a square, we can quickly calculate its area by finding the side length squared. It has a side length of 'c.' So, it's area is 'c' squared. Remember that the area of the large square is equal to the area of the four triangles plus the area of the small square. The area of the large square is 'a' squared plus '2ab' plus 'b' squared. The area of the four triangles is '2ab'... and the area of the small square is 'c' squared. Notice that '2ab' occurs on both sides of the equation. So, we can use the subtraction property of equality to subtract '2ab' from both sides. This leaves us with 'a' squared plus 'b' squared equals 'c' squared. A' and 'b' are the legs of a right triangle, and 'c' is the hypotenuse. This proves that the Pythaogrean Theorem is true for ALL right triangles. Let's review our proof of the Pythagorean Theorem. We started with a shape composed of a square within a square. We calculated the area of the large square and set it equal to the area of the shapes it is composed of. This resulted in the equation 'a' squared plus 'b' squared equals 'c' squared. Proving this gives us confidence that the Pythagorean Theorem is true for ANY right triangle. The Pythagorean Theorem can be used to determine an unknown side of a right triangle given two other sides. Now, if only Sina could help Maurice have a eureka moment for science!