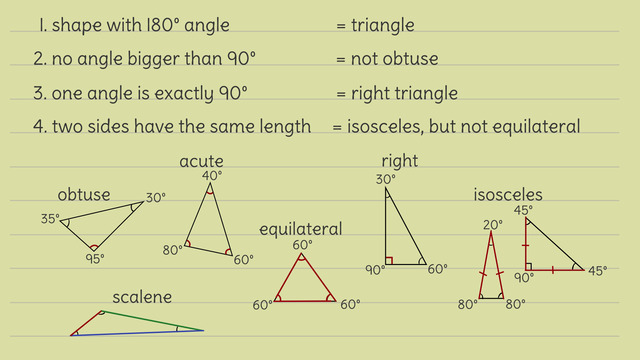
Trig Ratios in Right Triangles

Basics on the topic Trig Ratios in Right Triangles
To calculate unknown side lengths and non-right angles of right triangles, trigonometric ratios are the keys to your success.
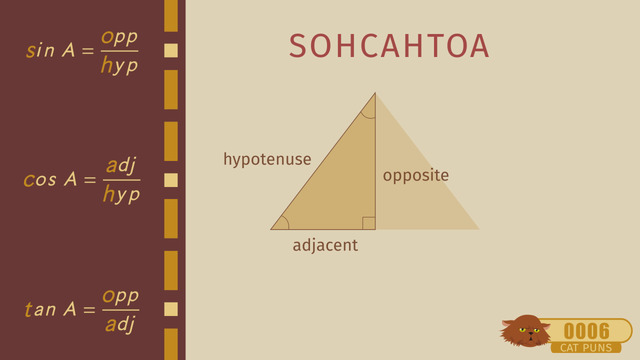
Just to refresh your memory, right triangles have a 90 degree angle. Look for the box in the corner to indicate a right angle. The side opposite the right angle is the hypotenuse, and the other sides are adjacent or opposite, depending on which angle you are considering.
The trig ratios you will use the most are sine, cosine, and tangent. Sine is the ratio of opposite to hypotenuse, cosine is the ratio of adjacent to hypotenuse, and tangent is the ratio of sine to cosine. Which ratio do you use to solve for an unknown measurement? That depends on what information is given in a problem. To help you solve problems, you’ll want to use a calculator that has the trig ratios or use a chart of the ratios.
Why do you study trig ratios? The ratios are very useful to solve real world problems. Before you hike up a steep mountain trail, do you want to know the distance of the trail? If you know the height of the mountain and one of the non-right angles, with the trig ratios you can calculate the measurement. Maybe you forgot to bring your calculator along on the hike – still it’s awesome to know that you can figure this out. To learn more, watch this video.
Define trigonometric ratios and solve problems involving right triangles
CCSS.MATH.CONTENT.HSG.SRT.C.6
Transcript Trig Ratios in Right Triangles
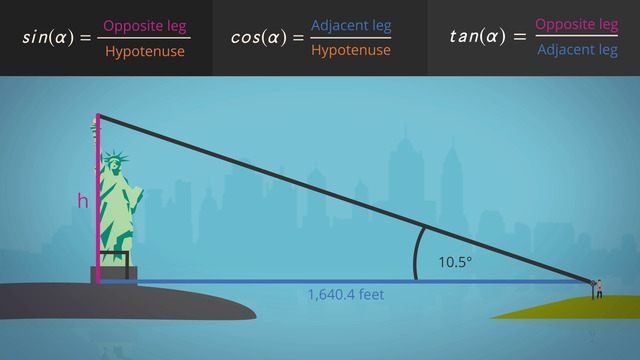
While visiting NYC, Kevin gets a look at the Statue of Liberty. He wonders, how tall is this lady?
How to calculate the Height of the Statue of Liberty
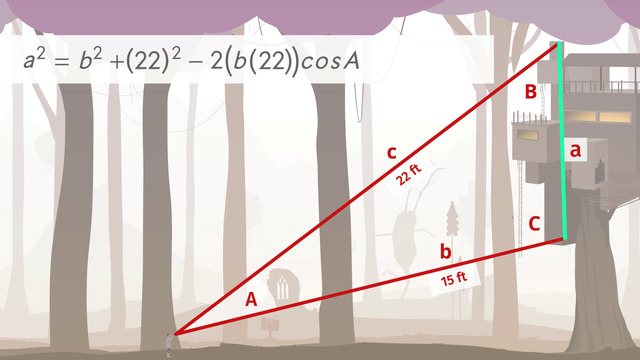
He looks through the viewing binoculars and notices the angle formed with the bottom of the base to the top of the statue's torch is ten and one-half degrees. How can Kevin use the trigonometry ratios of sine, cosine, and tangent of right triangles to calculate the unknown height of the Statue of Liberty?
Let's check out this situation. Can you see it builds a right triangle? Draw a line for the distance, then draw a line from the platform to the top of the triangle. From the viewfinder, we know the angle is ten point five degrees; write that in. Last draw in the line for the height. Don't forget to indicate the right angle.
Parts of a Right Triangle
Let's take a moment to review what we know about right triangles. The side opposite the right angle is named the hypotenuse, and the two sides adjacent to the right angle are the legs of the triangle. Across from angle alpha is the opposite side, and the adjacent side is between angle alpha and the right angle.
How to calculate sine, cosine, and tangent
To calculate the sine of angle alpha, sin(α), divide the length of the opposite leg by the length of the hypotenuse, and to find the cosine of angle alpha, cos(α), you divide the length of the adjacent leg by the length of the hypotenuse. For the tangent of angle alpha, tan(α), just divide the length of the opposite leg by the length of the adjacent leg.
Which Trigonometric Ratio should be used?
Which trigonometric ratio should you use to solve this problem? Sine, cosine, or tangent? Since you know the length of the adjacent leg, but not the hypotenuse, the tangent ratio is the best choice to calculate the length of the leg that is opposite angle alpha, the height of the Statue of Liberty.
Using the tangent ratio, set up the equation then just plug in the values you know. The tangent of 10.5 degrees times 1640.4 feet = the length of the opposite side.You will need to use your calculator to determine the tangent of 10.5 degrees, then multiply that number by 1640.4 feet to solve for the unknown height, the opposite leg.
She's a big one, 304 feet tall! Keven thinks trigonometric ratios are really fun and useful. The bird, on the other hand...
Trig Ratios in Right Triangles exercise
-
Find the trigonometric ratio that describes the angle of the given right triangle.
HintsThe naming of the opposite and adjacent legs depends on the position of $\theta$.
For every right triangle, there is only one correct ratio.
SolutionFor right triangles, there are basically three trigonometric ratios. Let's see what this means by looking at the pictured triangle.
The sine ratio is formed by dividing the opposite leg by the hypotenuse:
- $\sin (\theta) = \frac{a}{c}$
- $a$: opposite leg
- $c$: hypotenuse
- $\cos (\theta) = \frac{b}{c}$
- $b$: adjacent leg
- $c$: hypotenuse
- $\tan (\theta) = \frac{a}{b}$
- $a$: opposite leg
- $b$: adjacent leg
-
Evaluate the height of the Statue of Liberty using trigonometric ratios.
HintsEvery right triangle consists of a hypotenuse, opposite leg, and adjacent leg.
You can evaluate an angle by using one of the trigonometric ratios.
SolutionWe want to evaluate the height of the Statue of Liberty. But how can we do that?
In a right triangle, the hypotenuse is always across from the right angle. The adjacent leg, along with the hypotenuse form $\angle A$. The opposite leg is always across from $\angle A$.
The three most common trigonometric ratios are sine, cosine and tangent. Let's take a closer look:
- $\sin A = \frac{\text{opposite leg}}{\text{hypotenuse}}$
- $\cos A = \frac{\text{adjacent leg}}{\text{hypotenuse}}$
- $\tan A = \frac{\text{opposite leg}}{\text{adjacent leg}}$
The best trigonometric ratio to use is the tangent ratio. We want to evaluate the opposite leg, or the height of the Statue of Liberty, so we need to rearrange the equation. We can then plug these numbers into a calculator to solve for the $\tan A$:
$\begin{align} \tan A& = \frac{\text{opposite leg}}{\text{adjacent leg}}\\ \tan A \times \text{adjacent leg} &= \text{opposite leg}\\ \tan 10.5^\circ \times 1,640.4~\text{feet}&= \text{opposite leg}\\ 0.1853... \times 1,640.4~\text{feet}&= \text{opposite leg}\\ 304~\text{feet} &= \text{opposite leg} \end{align}$
Therefore, the height of the Statue of Liberty is $304$ feet.
-
Solve for the height of each tree.
HintsThe adjacent leg of the right triangle corresponds to the distance to each tree.
You are looking for the height of each tree, represented by the opposite leg of the right triangle.
The only trigonometric ratio that includes both opposite and adjacent legs is:
$\large{\tan A= \frac{\text{opposite leg}}{\text{adjacent leg}}}$
SolutionBy using the trigonometric ratios, Kevin can determine the height of each tree. First, he must decide which ratio will help him with the calculation.
He knows the approximate distance to each tree and the viewing angle, $\angle A$, for each tree.
Because the adjacent leg corresponds to the distance to each tree, and the opposite leg represents the height of each tree, the tangent ratio is the most useful to solve this problem.
$\tan A = \frac{\text{opposite leg}}{\text{adjacent leg}}$
In order to solve for the unknown height of the opposite leg, he modifies the equation. This is what he gets:
$\tan A \times \text{adjacent leg} = \text{opposite leg}$
Now he can plug in the given information. Let's solve for the height of the tallest tree in Kevin's garden, which stands at a distance of $164$ feet and an angle of $20.1^\circ$:
$\tan 20.1^\circ \times 164 \approx 60$
The tallest tree has a height of $60$ feet.
-
Determine the distance to the Eiffel Tower.
HintsWhich sides do you know? Which angle is given?
SolutionKevin is passionate traveler. Today he's in Paris. He remembers when he was in NYC he calculated the height of the Statue of Liberty, now that he's in Paris, he wants to calculate his distance from the Eiffel Tower.
He knows he can use one of the trigonometric ratios. From a guide book, he knows the height of the Eiffel Tower is $986$ feet. Thanks to his binoculars, he knows the viewing angle, $\angle A=20.6^\circ$. Considering the given information, he decides to use tangent to find out how far he is from the Eiffel Tower:
$\begin{align} \tan A & = \frac{\text{opposite leg}}{\text{adjacent leg}}\\ \tan A \times \text{adjacent leg} & = \text{opposite leg}\\ \text{adjacent leg} & = \frac{\text{opposite leg}}{\tan A}\\ \text{adjacent leg} & = \frac{986}{\tan 20.6^\circ}\\ & = 2,623.2 \end{align}$
Kevin is $2,623.2$ feet away from the Eiffel Tower.
-
Identify the properties of right triangles.
HintsThe hypotenuse is always the longest leg of a right triangle.
Adjacent leg and opposite leg depend on the position of $\angle A$.
SolutionUnderstanding the characteristics right triangles can teach us a lot about geometry.
Every right triangle has exactly one right angle. Two right angles are not possible. Try it out! (...but don't spend too much time trying.)
The side opposite the right angle is called the hypotenuse, and this is always the longest side of a right triangle. The opposite leg is across from $\angle A$, and the adjacent leg is always between $\angle A$ and the right angle.
As you maybe have noticed, you can change the position of $\angle A$. Then, the opposite leg becomes the adjacent leg, and the adjacent leg becomes the opposite leg. The sides are always named according to the location of angle $A$.
-
Calculate the side lengths and angles of the right triangle.
HintsDid you know that the interior angles of every triangle add up to the same number?
$\angle A + \angle B + \angle C = 180^\circ$
SolutionWe want to find the missing information in order to complete our right triangle. We know that $\angle A = 37^\circ$ and that the hypotenuse has a length of $5$ feet.
The given information is enough to calculate what we don't know. Also, there are several ways to solve this problem.
First, we can find $\angle B$ since we know a triangle's internal angles sum to $180^\circ$.
$\begin{align} \\ 180^\circ - \angle A - \angle C &= \angle B \\ 180^\circ - 37^\circ - 90^\circ &= \angle B \\ 53^\circ &= \angle B \end{align}$.
Now, we can use either the sine or cosine ratios to solve for the unknown side measures. Let's use cosine first. The correct ratio for cosine is:
$\cos B = \frac{\text{adjacent leg}}{\text{hypotenuse}}$
When we plug in our values, this is what we get:
$\begin{align} \cos 53^\circ & = \frac{a}{5}\\ \cos 53^\circ \times 5 & = a\\ 4 & \approx a \end{align}$
Now we have all lengths except for side $b$. Let's use the sine ratio to calculate the length of side $b$.
$\sin B = \frac{\text{opposite leg}}{\text{hypotenuse}}$
$\begin{align} \sin 53^\circ & = \frac{b}{5}\\ \sin 53^\circ \times 5 & = b\\ 3 & \approx b \end{align}$
Side $b$ has a length of $3$ feet.