Exponents
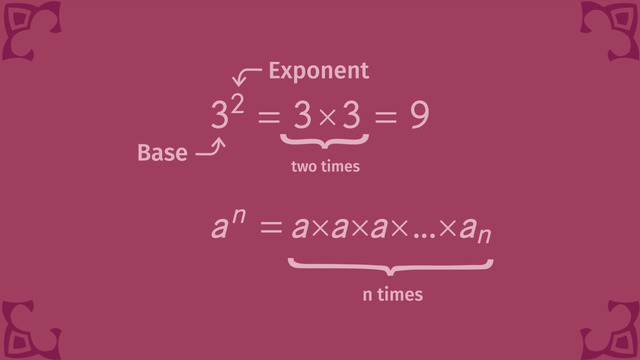

Basics on the topic Exponents
An exponent is shorthand for the repeated multiplication of a certain number by itself. In exponential notation an, a is the base while n is the exponent. The base a is the number which is multiplied n times by itself. For example, instead of writing the very long multiplication expression 2222222*2, we can write it as 28. In this example, 2 is the base while 8 is the exponent. Simplifying 28 further gives us 256.
Taking the exponent of a number means raising it to a power where the exponent is the power. The exponential notation 54 means raising 5 to the fourth power. A number raised to the second power is said to be squared. Thus, 52 is read as 5 squared. On the other hand, a number raised to the third power is said to be cubed. Thus, 43 is read as 4 cubed. Incidentally, a perfect square is a number which can be expressed as a square of a certain number while a perfect cube is a number which can be expressed as a cube of a certain number. The number 9 is a perfect square because it is equal to 32 while 125 is a perfect cube because it is equal to 53.
Some other interesting properties of exponents are that any base raised to 1 is equal to itself and any base raised to zero is equal to 1. Thus, 81 equals 8 while 90 equals 1.
Be very careful when entering into any agreement involving money or assets used in exponential notations as we must never underestimate the power of exponential growth.
Work with radicals and integer exponents.
CCSS.MATH.CONTENT.8.EE.A.1
Transcript Exponents
Once upon a time in a city in Persia, Maharaja Maini lived in a beautiful and ostentatious royal palace. One day, he realizes that his vault is overflowing with gold coins. He decides that he needs a larger vault to house his considerable treasure, and of course a greedy Maharaja like him wants to find the cheapest architect to work on his project. One offer in particular piques his interest. For payment, the architect requests 2 gold coins on the first day, 4 on the second, 8 on the third and so on. The payment continues to double each day until 30 days have passed. This seems to be a very good offer but the Maharaja's about to get a lesson in exponents. Let's take a look at how this payment system will unfold.
The architect gets paid daily. On the first day, the Maharaja pays the architect 2 gold coins. On the second day, he's gotta fork over double what he paid the previous day, for a total of 4 coins. By the 5th day, the Maharaja begins to wonder if his choice really was a good one.
The Maharaja decides he should calculate the payment for the rest of the month. He then starts calculating the payment for each day on a calendar. Hm...there isn't enough room in the box to write out what the Maharaja owes the architect on the 6th day.
Could there be a better way to write this? Let's see if we can find a pattern. You probably already know that multiplication is just repeated addition. For example, you already know a shorter way to write 2 plus 2 plus 2 plus 2 plus 2 plus 2. You can simply write 6 times 2. Both expressions equal 12. But, is there a shorter way to write repeated multiplication? There is! For example, we could write 2 times 2 times 2 times 2 times 2 times 2 as 2 to the sixth power which gives us 64.
Exponents offer a shorter way to write the repeated multiplcation of a single number. For example, let's take a look at 3 squared. In this example, 3 is our base and we call 2 the exponent, or power. The exponent represents the number of times a number is multiplied by itself. The expanded version of 3 to the 2nd power is 3 times 3 which equals 9, so 3 squared is 9. The typical way to express a number in general exponential form is 'a' to the nth power. This is the same as 'a' times 'a' times 'a' times 'a', 'n' times. Now the Maharaja can write what he owes the architect in the space provided on his calendar!
Let's see if we can rewrite what the Maharaja owes the architect for the first 5 days. On day 1, the Maharaja owes the architect 2 gold coins which we can write this as 2 to the first power. On day 2, the Maharaja owes the architect 2 squared gold coins. On day 3, the Maharaja owes the architect 2 cubed gold coins. Are you sensing a pattern? How could we express how many gold coins the Maharaja owes the architect on day 5? That's right! We can write it as 2 to the 5th power!
By the 30th day, Maharaja Maini, using 2 as his base and 30 as his exponent calculates what he owes the architect on day 30. After paying the architect, Maharaja Maini senses there must be a mistake his vault is expanded, just like he wanted. But now the Maharaja's got a new problem.
Exponents exercise
-
Explain the expression $2^6$.
HintsThere exists a shorter way to write $4+4+4+4+4=5\times 4=20$. Repeated addition is just multiplication. So what is repeated multiplication?
You can understand the expressions depending on their position:
- $2$ is in the bottom.
- $6$ is in the top.
$3^4=3\times 3\times 3\times 3=81$.
Remember the following notation:
factor $\times$ factor $=$ product.
SolutionYou know the shorter way to write
$2+2+2+2+2+2=6\times 2=12$.
Does there also exist a shorter way to write repeated multiplication?
Yes!
We can write $2\times 2\times 2\times 2\times 2\times 2$ as $2^6$, and both equal $64$.
$2^6$ is read as "two to the sixth power".
- $2$ is the base.
- $6$ is the exponent.
When expanding exponents, the factor is written as many times as the exponents state. So here $2^6=2\times 2\times 2\times 2\times 2\times 2=64$. The factor $2$ is written six times in the product.
-
Find the right expression for the given example.
Hints- Day $1$: $2$ coins, or in exponential form $2^1$.
- Day $2$: $4$ coins, as factors $2 \times 2$, or in exponential form as $2^2$.
- Day $3$: $8$ coins, as factors $2 \times 2 \times 2$, or in exponential form $2^3$.
- Day $4$: $16$ coins, as factors $2 \times 2 \times 2 \times 2 $, or in exponential form $2^4$.
- Day $5$: $32$ coins, as factors $2 \times 2 \times 2 \times 2 \times 2$, or in exponential form $2^5$.
Each of the golden coins can be written with base $2$ because they're always doubled.
SolutionLet's examine the payment depending on the number of days:
- Day $1$: $2$ coins, or in exponential form $2^1$.
- Day $2$: $4$ coins, as factors $2 \times 2$, or in exponential form as $2^2$.
- Day $3$: $8$ coins, as factors $2 \times 2 \times 2$, or in exponential form $2^3$.
- Day $4$: $16$ coins, as factors $2 \times 2 \times 2 \times 2 $, or in exponential form $2^4$.
- Day $5$: $32$ coins, as factors $2 \times 2 \times 2 \times 2 \times 2$, or in exponential form $2^5$.
Thus we can conclude the payment on day 30 is $2^{30}=1,073,741,824$ gold coins.
Perhaps the deal wasn't as profitable for the Maharaja as he thought.
-
Determine the expression describing the total number of cats.
HintsExamine the base and the exponent of each expression.
For example,
$4^4=4\times 4\times 4\times 4$.
This product contains the factor $4$ four times.
SolutionLisa, Ben and John are three people.
Each of them has three friends. We can multiply $3$ by $3$: three friends for each of the three people.
So there are $3\times 3=3^2=9$ people in total.
Each of them has three cats. Thus we should multiply the number of friends $3^2$ once again by $3$. This is $3\times 3\times 3=3^3$, or $3$ to the third power.
We can simplify the exponential form to $3^3=27$.
This is the total number of cats.
-
Transform the given expression into exponential form.
HintsThe exponential form of a product with $n$ times the same factor is the short-hand way of writing the repeated multiplication.
$5\times5\times5=5^3$
Count the frequency of the factor.
The repeating factor is the base of the exponential form and the frequency the exponent.
SolutionWe write a product with $n$ times the same factor as a power:
$a^n=\underbrace{a\times a\times a...\times a}_{n~\text{times}}$.
We have to consider the factor $a$, which is the base, and the frequency of repeating multiplication $n$, which is the exponent.
Let's look at some examples:
- $4\times4\times 4\times 4\times4$: here we have a product with five times the factor $4$. Thus $4$ is the base, while $5$ is the exponent: $4^5$.
- Where as, with $5\times5\times 5\times 5$ we have a product with four times the factor $5$. Thus $5$ is the base, while $4$ is the exponent: $5^4$.
- $7\times7\times 7$. Here we have a product with three times the factor $7$. This gives us the corresponding power $7^3$.
- Be cautious: with $3\times3\times 3\times 3\times 3\times 3\times 3$, we have a product with seven times the factor $3$. Thus, $3$ is the base, while $7$ is the exponent: $3^7$.
- $6\times 6=6^2$
- $2\times 2\times 2\times 2\times 2\times 2=2^6$
-
Name the parts of the exponential expression $3^2$.
HintsIf you have a fraction, like $\frac ND$, then $D$ is called the denominator.
In this expression $4^7$, the $7$ represents the exponent.
SolutionThe main theme of this exercise is the exponent, which is a part of a power. An example for a power is $3^2$.
It's short-hand notation for writing a product with twice the factor $3$; so we have $3^2=3\times 3=9$. It's not $3\times 2=6$, which is short-hand notation for a sum with twice the summand $3$.
The whole expression $3^2$ is called a power, namely $3$ to the second power.
- $3$ is the base.
- $2$ is the exponent.
-
Calculate the given expressions.
HintsYou can compare two powers with the same base: $2^3<2^4$, because $3<4$.
You can also compare powers with the same exponents without calculating the values: $3^4<5^4$, because $3<5$.
If neither the base nor the exponent agree you have to calculate the values.
For this keep in mind, that the power is a short writing for a repeating multiplication. For example, $5^3=5\times 5\times 5$.
SolutionIf you'd like to compare powers, the best way is to calculate the regarding values. For this keep in mind that
$a^n=\underbrace{a\times a\times a...\times a}_{n~\text{times}}$.
- $2^3=2\times 2\times 2=8$
- $3^2=3\times3=9$
- $4^2=4\times 4=16$
- $5^2=5\times 5=25$
- $3^3=3\times 3\times 3=27$
- $2^5=2\times 2\times 2\times 2\times 2=32$
- $6^2=6\times 6=36$
- $4^3=4\times 4\times 4=64$











