Ratio and Proportions
Basics on the topic Ratio and Proportions
Ratios are used to compare quantities, and two equal ratios make a proportion.
You can represent ratios in three different ways – separating the quantities with the word to, separating the quantities with a colon, and writing the two quantities as a fraction. Like a fraction, you can reduce a ratio to its lowest terms by dividing both quantities by their greatest common factor.
If there are 9 apples for every 12 apples in a fruit basket, the ratio is 9 to 12, or reduced to lowest terms, 3 to 4. You can change the order and still model the same information, 12 to 9 which is reduced to 4 to 3. When using ratios and proportions to solve problems, just make sure to be consistent in the ordering of the quantities.
What kinds of problems real world problems can you use ratios and proportions to solve? Party planning, food supply, manufacturing – the list is pretty much endless. When quantities have a proportional relationship, using equal ratios to create a proportion and then using algebra equations to solve for any unknown quantities is a great strategy to solve problems. For every video you watch you will have a ton of fun. What’s the ratio? 1 video : tons of fun.
Analyze proportional relationships and use them to solve real-world and mathematical problems.
CCSS.MATH.CONTENT.7.RP.A.1
Transcript Ratio and Proportions
Dr. Evil is very excited. Tonight, he’s going to a speed dating event. He's hoping to meet the future Mrs. Evil.
What are Ratios & Proportions?
To start the day, he makes an evil smoothie. Dr. Evil uses his knowledge of ratios and proportions to create the perfect concoction. Ratios are used to compare quantities, and two equal ratios create a proportion.
Writing a Ratio (Example 1)
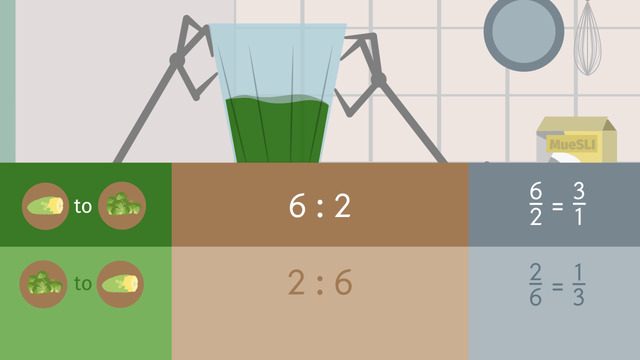
He adds six parts Monstera Deliciosas to two parts brussels sprouts. FYI: Monstera Deliciosa is a tasty tropical fruit. Imagine a banana-pineapple hybrid! So he has a ratio of six parts Monstera Deliciosas to two parts brussels sprouts.
We can write this ratio in three different formats. We can use the word to, a colon, or a fraction.
It's best to reduce ratios to their lowest terms. So for this situation, the ratio of Monstera Deliciosas to brussels sprouts reduced to lowest terms is three to one.
We can reverse the order of the ratio and still provide the same information. Flip the ratio, and we get brussels sprouts to Monster Deliciosas, a ratio of two to six, which reduces to one to three.
Be careful to match up the quantities with their proper labels. Imagine 6 parts brussels sprouts to only 2 parts Monster Deliciosas, yuck!
Two Equal Ratios Create a Proportion (Example 2)
After slurping down the smoothie, Dr. Evil works on his latest project, a new, evil hair potion for men. He decides to try it out on himself, as he wants to look especially evil for the speed dating event.
He uses one drop of the potion and voilà, he can style his hair in five different ways but only one out five of the hair styles looks evil.
We can write this as a ratio, one to five. He does a second test, this time using two drops of the potion. The result is now two out of the ten styles look evil. As a ratio, this is two to ten and in reduced terms, one to five.
What's going on here? The two ratios reduce to the same ratio. That’s right, the two ratios are equal, meaning the quantities are proportional. Two equal ratios create a proportion.
Example 3
At the speed dating event, Dr Evil knows it’s a numbers game. In the past, two to eight women were interested in him.
Two to eight is proportional to one to four. There are four women at the event, so if all goes according to plan, he will match with one very evil lady!
Dr. Evil, really? That's your special lady? Well. As the saying goes, 'Beauty is in the eye of the beholder.’
Ratio and Proportions exercise
-
Explain the ratio of the ingredients for Dr. Evil's smoothie.
HintsImagine the monstera deliciosa being on one side and the Brussels sprouts on the other side.
Now write the number of parts below each ingredient and put a $:$ sign in between.
To reduce a fraction, we divide the numerator and the denominator by the same number.
Take a look at the following example:
$\frac8{12}=\frac{8\div 4}{12\div 4}=\frac23$.
SolutionWe know that Dr. Evil needs six parts of monstera deliciosa and only two parts of Brussels sprouts for his smoothie. This can be written as following:
$\begin{array}{ccc} \text{monstera deliciosa }&&\text{Brussels sprouts}\\ 6&:&2 \end{array}$
This is the ratio, and it can also be written in fraction form:
$\frac62=\frac{6\div2}{2\div2}=\frac31$.
Now we change the order to the ratio of Brussels sprouts to monstera deliciosa.
$\begin{array}{ccc} \text{brussels sprouts}&&\text{monstera deliciosa }\\ 2&:&6 \end{array}$
This is the ratio. Written in fraction form:
$\frac26=\frac{2\div2}{6\div2}=\frac13$.
-
Determine how many women are interested in Dr. Evil.
HintsThe ratio of women interested in Dr. Evil compared to all women is equal to $2:8$.
Write $2:8$ as a fraction then reduce it to lowest terms.
If the fraction is given by $\frac ?4$ you can find the answer in the numerator.
SolutionDr. Evil knows that two out of eight women will be interested in him. This can be written as $2:8$.
To calculate other combinations, we can write this as a equivalent fraction and fill in the unknown values:
$\frac28=\frac{2\div 2}{8\div 2}=\frac14$.
Now we also know that one out of four women will be interested in Dr. Evil.
So if he meets $4$ women, $1$ of them will be interested in him.
-
Determine the right ratio.
HintsReduce each fraction to its lowest terms.
You can reduce a fraction by dividing the numerator and the denominator by the same number.
Take a look at the following example:
$\frac{121}{22}=\frac{121\div11}{22\div11}=\frac{11}2$
SolutionTo decide which fraction belongs to which ratio, we have to reduce each fraction to its lowest terms:
- $\frac{10}{15}=\frac{10\div5}{15\div5}=\frac23$ $\rightarrow 2:3$
- $\frac{22}{33}=\frac{22\div11}{33\div11}=\frac23$ $\rightarrow 2:3$
- $\frac{4}{6}=\frac{4\div2}{6\div2}=\frac23$ $\rightarrow 2:3$
- $\frac{50}{60}=\frac{50\div10}{60\div10}=\frac56$ $\rightarrow 5:6$
- $\frac{15}{18}=\frac{15\div3}{18\div3}=\frac56$ $\rightarrow 5:6$
- $\frac{49}{63}=\frac{49\div7}{63\div7}=\frac79$ $\rightarrow 7:9$
- $\frac{21}{27}=\frac{21\div3}{27\div3}=\frac79$ $\rightarrow 7:9$
-
Determine the corresponding ratios.
HintsBe careful with the order of the ratio.
Write the given ratio as a fraction and reduce the fraction as much as possible.
SolutionFor each relation we write the ratio as a fraction and reduce this fraction to its lowest terms.
- Dragon blood to tear drops: $\frac5{15}=\frac{5\div5}{15\div 5}=\frac13~\rightarrow~1:3$
- Snake poison to water: $\frac{20}{35}=\frac{20\div5}{35\div 5}=\frac47~\rightarrow~4:7$
- Water to dragon blood: $\frac{35}{5}=\frac{35\div5}{5\div 5}=\frac71~\rightarrow~7:1$
- Tear drops to snake poison: $\frac{15}{20}=\frac{15\div5}{20\div 5}=\frac34~\rightarrow~3:4$
- Water to snake poison: $\frac{35}{20}=\frac{35\div5}{20\div 5}=\frac74~\rightarrow~7:4$
- Snake poison to tear drops: $\frac{20}{15}=\frac{20\div5}{15\div 5}=\frac43~\rightarrow~4:3$
-
Describe the difference between ratio and proportion.
Hints$6:2$ and $3:1$ are both ratios.
A proportion is like an equation.
SolutionKeep in mind:
Ratios are used to compare quantities.
Two equal ratios create a proportion.
For example:
- The ratio is $6:2$ or $3:1$.
- The proportion is $\frac 62 = \frac 31$.
-
Calculate the number of ingredients for 30 stinging nettle plants.
HintsThe ratio stinging nettle to cod liver oil is $3:1$.
Write this ratio as a fraction then write an equivalent fraction with a numerator equal to $30$.
Then you can solve for the unknown denominator.
The ingredients in increasing order:
- cod liver oil
- stinging nettle
- chamomile
- Artemisia
The total number of cod liver oil, stinging nettle, and chamomile plants is 100.
SolutionWe know that the number of stinging nettle plants is $30$.
Let's begin with cod liver oil: The number of stinging nettle plants is three times the number of the cod liver oil. That means $3:1$. We write this ratio as a fraction and raise the terms by $10$:
$\frac 31=\frac{3\times 10}{1\times 10}=\frac{30}{10}$.
- In the numerator we have the number of stinging nettle plants: $30$.
- The denominator shows the number of the cod liver oil: $10$.
We can write this as a fraction $\frac12$ and raise the terms by $30$:
$\frac 12=\frac{1\times 30}{2\times 30}=\frac{30}{60}$.
- In the numerator we have the number of stinging nettle plants: $30$
- The denominator shows the number of the chamomile plants: $60$
Finally, we calculate the number of Artemisia plants: the number of Artemisia plants must always be twice as much as the number of all other ingredients. This is given by $2:1$. Let's write it as a fraction and raise the terms by $100$, the total number of different plants:
$\frac 21=\frac{2\times 100}{1\times 100}=\frac{200}{100}$.
- In the denominator we have the total number of all other plants: $100$.
- The numerator shows the number of artemisia plants: $200$.