Solving Quadratic Equations by Completing the Square
Basics on the topic Solving Quadratic Equations by Completing the Square
One of the most effective methods of solving a quadratic equation is by completing the square. It is also the method that makes it easiest to graph the equation as well.
By completing the square, we can find, not only the roots of the equation, but also the vertex of the graph of the equation. This is because we will pass from the standard form, ax2 + bx + c = 0, to the vertex form, a (x - h)2 + k = 0, with (h,k) as the vertex of the graph.
The steps of this method must be taken carefully, though. The standard form must be gradually solved to arrive at the vertex form: 1. Finding the value that needs to be added on both sides of the equation to complete the square; 2. Finding the square roots of both sides of the equation; 3. Finding the roots of the equation by finally solving for x using the positive and negative values of the square root of the right side.
In this video, the method of completing the square to solve an equation and plotting its graph is explained.
Analyze Functions Using Different Representations.
CCSS.MATH.CONTENT.HSF.IF.C.8.A
Transcript Solving Quadratic Equations by Completing the Square
Kata Ana is always late for ninja school. Luckily, she’s had a lot of practice finding the quickest way to her school. Kata needs to know how to solve quadratic equations by completing the square in order to avoid some of the dangerous obstacles on her way to school. Kata has to use her grappling hook to swing over to the lantern over there. She throws her grappling hook onto the school’s roof. Kata knows that she has to avoid the school's guard Komodo dragon. She uses a technique passed down for generations called completing the square.
Kata knows that if she does this, she can find the zeros of any parabola as well as the parabola’s vertex by putting the standard form of the quadratic formula, ax² + bx + c = 0 into vertex form, a(x - h)² + k = 0. Completing the Square certainly is a powerful tool.
Vertex Form
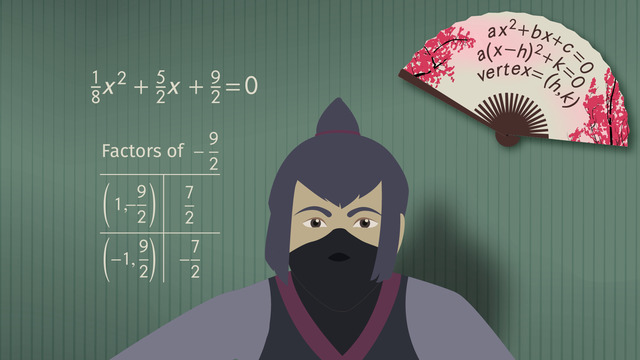
The first equation Kata has is one-eighth x² plus five over two 'x' plus nine over two equals 0. Kata looks at this problem. She tries to list the factors of negative nine over two: 1, negative nine over two and -1, nine over two. But neither of these options sum to five over two. Kata needs a more powerful tool. To complete the square, Kata needs to get the equation in vertex form. First, she moves the nine over two to the right hand side using opposite operations. This leaves her with one-eighth x² plus five over two 'x' equals negative nine over two.
Next, she removes one-eighth from the equation by dividing each coefficient by one-eighth, leaving her with one-eighth times the quantity 'x' squared plus twenty 'x' equals negative nine over two. Now she takes half of her ‘b’ term, 20, giving her 10. She then squares the 10 and needs to add the result on both sides of the equation. However, since this part of the equation is inside the parentheses, and the contents of the parentheses is multiplied by one-eighth, we have to add 100 times one-eighth on the right side of the equation. We should simplify the fractions on the right side of the equation as much as possible.
Kata notices that the left side of the equation is now a perfect square trinomial, which she knows how to factor. All Kata has to do now is move the 8 to the left side of the equation and compare her result to the standard vertex form of the equation to figure out the signs of the vertex. One-eighth times (x + 10)² minus 8= 0.Since the signs inside the parentheses don't match, we have to change 10 to negative 10. The signs are also different for the constant, so we have to change 8 to negative 8. The vertex has revealed itself! The vertex is (-10, -8)!
Finding the Zeros by Isolating x
Now, to get the zeros of the parabola!
Let's start by getting rid of the one-eighth on the left side of the equation. We can do this by multiplying both sides of the equation by 8, giving us 'x' plus 10 quantity squared equals 64. Now we can take the square root of both sides. We're left with 'x' plus 10 equals plus/minus 8. For the last step, we isolate the 'x' and get our solutions, negative 2 and negative 18!
Completing the Square really is a powerful tool. Kata Ana can clearly see why her ancestors have been using it for generations!
Second Example
Kata now needs to be able to clear that wall without hitting the roof so she can land safely on the ledge of her school. The equation for this part is negative one-tenth x² + eight-fifths 'x' plus 18-fifths equals zero. For this equation, Kata Ana repeats the process she used to solve the first equation. She first moves the constant to the right-hand side. Then, she divides the left side of the equation by negative one-tenth.
Again, she takes half of her ‘b’ term, -16, giving her -8, which she then squares and adds to both sides of the equation. She remembers that, since the negative one-tenth is outside the parentheses, she has to multiply 64 by one-tenth before adding it to the right side of the equation. The left side of the equation is a perfect square trinomial! Completing the Square never fails!
Using opposite operations, Kata rewrites the left side of the equation as negative one-tenth times the quantity 'x' minus 8, squared plus 10. Great, now the equation is in standard form. Once again, we compare the signs. Can you tell what the vertex is? That's right! it's at (8, 10)! Once again, to get the zeros of the parabola! Taking the square root on both sides of the equation leaves 'x' minus eight equal to plus/minus 10. And finally, isolating the variable gives us our solutions. Thanks to her knowledge of completing the square and some quick thinking, Kata is able to land safely on the ledge of her school.
What’s this?! What's happened to her classmates?! Kata Ana is Shell Shocked!
Solving Quadratic Equations by Completing the Square exercise
-
Determine the vertex of the parabola.
HintsWhat is the format of a perfect square trinomial? You will need to add a term to the expression inside the parentheses on the left hand side of the equation in order to turn it into a perfect square trinomial.
For an expression in the form $ax^2 + bx$, the third term that must be added to make it a perfect square trinomial is of the form: $(\frac{b}{2})^2$.
Remember that the vertex form of the equation is $a (x-h)^2 + k = 0$. Pay close attention to the signs when you are determining the values of $h$ and $k$.
SolutionKata Ana knows that if she rearranges the function into vertex form, she will be able to easily identify the coordinates of the vertex. Vertex form is $a(x-h)^2+k = 0$, and gives the vertex as $(h,k)$.
If the given equation was in standard form, she would begin by moving the constant term to the right hand side of the equation. Then she would factor out the coefficient on the $x^2$ term to give an expression in parentheses on the left hand side. She notices that this has already been done to the given equation, $\frac{1}{8}(x^2+20x)=-\frac{9}{2}$.
She knows that she now needs to get the left hand side of the given function into a form that includes a perfect square trinomial. She knows that this trinomial is the sum of three terms: a term with $x^2$, another with $x$, and a constant. The expression on the left hand side of the given function is missing the constant term. Kata Ana knows that she must determine what constant term should be added to result in an expression that is a perfect square. She can find this constant by taking the coefficient of the $x$ term, $20$, dividing it by two, and then squaring it. She finds $(\frac{20}{2})^2 = 10^2$.
She wants to add this term to the expression that is inside the parentheses on the left hand side. However, she knows that this entire expression in parentheses is being multiplied by $\frac{1}{8}$. So she really needs to add $\frac{1}{8}\times10^2$ to both sides, in order to add $10^2$ inside the parentheses on the left hand side.
$\begin{array}{rclcl} \frac{1}{8}(x^2+20x+10^2) & = & -\frac{9}{2}+\frac{10^2}{8} & | & \text{simplifying} \\ \frac{1}{8}(x^2+20x+10^2) & = & 8 \\ \end{array}$
Now she has a perfect square trionomial on the left hand side, $(x^2+20x+10^2)$. She can factor this trinomial to result in the following function:
$\frac{1}{8}(x+10)^2 = 8$.
Now, she can use opposite operations to get the function into vertex form:
$\frac{1}{8}(x+10)^2 -8 = 0$.
This shows her that $h = -10$, and $k=-8$. Therefore the vertex coordinates are $(-10,-8)$.
-
Find the zeroes of the quadratic equation.
HintsRemember that a positive or negative number can result from taking the square root of a given number.
You must pay close attention to signs.
SolutionKata Ana knows that she must find the zeroes, or roots, of the quadratic function. To do this, she must isolate the variable $x$.
She starts by observing that the given equation is in vertex form, which is $a (x-h)^2 + k = 0$. Next she uses opposite operations to move $k$ over to the the right hand side, and then divides both sides by $a$.
$ \begin{array}{rclcl} -\frac{1}{10}(x-8)^2 + 10 &=& 0&|&\text{subtract 10 from both sides} \\ -\frac{1}{10}(x-8)^2 &=&-10 &|&\text{divide by -1/10} \\ (x-8)^2 &=&100&|&\text{divide by -1/10} \\ \end{array} $
To continue to isolate $x$, she must take the square root of both sides of the equation. When taking the square root of a number, it is important to remember that the answer can be positive or negative. For example $\sqrt9 = \pm 3$ because $9=3^3=(-3)^2$. In this case:
$x-8=\pm 10$.
She then finishes isolating $x$, and arrives at:
$x = 8 \pm 10$.
She can now find the zeroes of the function to be:
$8 - 10 = -2$
and
$8 + 10 = 18$.
-
Solve for the zeroes of each quadratic equation.
HintsYou can find the zeroes by isolating $x$.
The given functions are in the form $a (x-h)^2 + k = 0$. How can you move $k$ and $a$ to the right hand side?
Remember that when you take the square root of a number, there are two possible answers. For example $\sqrt 81 = \pm9$.
SolutionThese functions have already been set equal to zero. The pair of roots for each function can therefore be found by isolating $x$.
For the first function:
$2(x-5)^2-8=0$.
We move the constant to the right hand side:
$2(x-5)^2=8$.
Then we can divide through by the term that is outside the parentheses on the left hand side:
$(x-5)^2=\dfrac{8}{2}=4$.
Then we can take the square root of each side:
$x-5=\sqrt4$.
Remember that the square root can be a positive or negative value:
$x-5=\pm 2$.
Finally, we can move the constant to the right hand side, and determine the roots:
$x=5 \pm 2$
$\begin{array}{rcl} x=5+2 & or & x=5-2 \\ x=7 & or & x=3 \end{array}$
So we find that the roots are $3$ and $7$.
Similarly, for the other equations, we find the roots:
$\begin{array}{rcl} -3(x+2)^2+27=0 & | & \text{roots are -5 and 1} \\ 4(x-2)^2-64=0 & | & \text{roots are -2 and 6} \\ -3(x-9)^2+12=0 & | & \text{roots are 7 and 11} \\ \end{array}$
-
Calculate the vertex and zeroes of the quadratic equation.
HintsIt will be helpful if you always reduce rational numbers to their simplest form during your solution.
At every step of the way, every term in this problem (both coefficients and constants) can be simplified to an integer. If you have a rational number that you can't immediately simplify to an integer in your solution, you should double check your calculations.
Remember, you can convert the standard form equation to vertex form by completing the square. The vertex will then be at the coordinates $(h,k)$.
Once you have the function in vertex form, you can solve for the zeroes by setting the function equal to zero, and isolating $x$.
There are two roots for this function.
SolutionWe need to find the vertex, and the roots of this function. We can find the vertex by converting the function into vertex form, $a (x-h)^2 + k = 0$.
We have the standard form function:
$-2x^2+24x-64=0$.
We start by moving the constant term to the right hand side:
$-2x^2+24x=64$.
Next, we factor out the coefficient on the $x^2$ term from the left hand side:
$-2(x^2-12x)=64$.
Then we take the coefficient on the $x$ term, divide it by two, and square the result:
$(\frac{12}{2})^2 = 6^2 = 36$.
Adding this constant to the expression inside the parentheses on the left hand side of the equation will give us a perfect square trinomial, which we know how to factor. In order to add the constant inside the parentheses on the left hand side, we have to add the constant times negative two on the right hand side. This is because all terms inside the parentheses on the left hand side are multiplied by negative two. So really, we add $36\times-2$ to both sides.
$ \begin{array}{rclcl} -2(x^2-12x+36) & = & 64+(36\times-2) &|&\text{Simplifying} \\ -2(x^2-12x+36) & = & 64-72 \\ -2(x^2-12x+36) & = & -8 \end{array} $
Now we can factor the perfect square trinomial on the left hand side, to get:
$-2(x-6)^2 = -8$.
And finally, we can move the constant to the left hand side, to get the equation in vertex form:
$-2(x-6)^2+8=0$.
This tells us that the vertex is at $(6,8)$.
Now, we can isolate for $x$ to find the roots.
The first step is to move the constant to the right hand side, which we have already done in an earlier step: $-2(x-6)^2 = -8$.
Then we can divide through by the term that is outside the parentheses on the left hand side:
$ \begin{array}{rclcl} (x-6)^2 & = & \frac{-8}{-2} &|&\text{simplifying} \\ (x-6)^2 & = & 4 \end{array} $
Taking the sqaure root of both sides is the next step to isolate $x$:
$x-6 = \sqrt4$.
This gives us two possible solutions, a positive one and a negative one:
$x-6 = \pm 2$.
We can then find the two roots of the function:
$\begin{array}{rcl} x=6+2 & or & x=6-2 \\ x=8 & or & x=4 \end{array}$
-
Identify the vertex form of a quadratic function.
HintsRemember that the standard form is the most simplified form. This is not the vertex form.
Remember that the vertex takes its name from the fact that it includes the coordinates of the parabola's vertex.
The vertex form gives the coordinates of the vertex as $(h,k)$.
SolutionThe standard form of a quadratic functon is $ax^2+bx+c=0$. This is the most simplified format, and is a trinomial.
The vertex form is $a (x-h)^2 + k = 0$. It reveals the coordinates of the vertex, which are $(h,k)$.
The factored form of a quadratic function is $a(x-p)(x-q)=0$. It shows the intercepts, which are $p$ and $q$.
$ax^2+bx=-c$ is a rearranged version of the standard form. It shows the first step in converting a standard form function into the vertex form. But it is not yet in the vertex form.
-
Identify the graphs of the functions.
HintsThe equations are given in vertex form.
Pay close attention to the signs.
How can you determine the coordinates of the vertex from the values $h$ and $k$ in a vertex form equation?
It may help to start by finding the correct vertices of each given equation. Then you can compare those vertices to those on the graph.
SolutionWe are given the vertices of three parabolas, and many quadratic functions. The quadratic functions are in vertex form.
We know that in general, the vertex form is:
$a (x-h)^2 + k = 0$,
Where the vertex is $(h,k)$.
Let's find the values of $h$ and $k$ that correspond to the vertex that is given for each parabola.
For the purple parabola, the vertex is given as $(2,-4)$. Therefore $h = 2$ and $k = -4$. Substituting these values into the vertex form of a quadratic function, we get:
$a (x-2)^2 -4 = 0$.
We don't yet know the value of $a$. The only function that is listed that matches the values that we found for $h$ and $k$ is:
$2(x-2)^2-4=0$.
Therefore this is the function that describes the purple parabola.
Similarly, for the blue parabola, we know the vertex is at $(4,12)$, which means that $h = 4$ and $k = -12$. The only given function that matches is:
$-3(x-4)^2+12=0$.
For the gold parabola, we know the vertex is at $(-1,-4)$, which means that $h = -1$ and $k = -4$. The only given function that matches is $(x+1)^2-4=0$.

What are Quadratic Functions?
Graphing Quadratic Functions
FOILing and Explanation for FOIL
Solving Quadratic Equations by Taking Square Roots
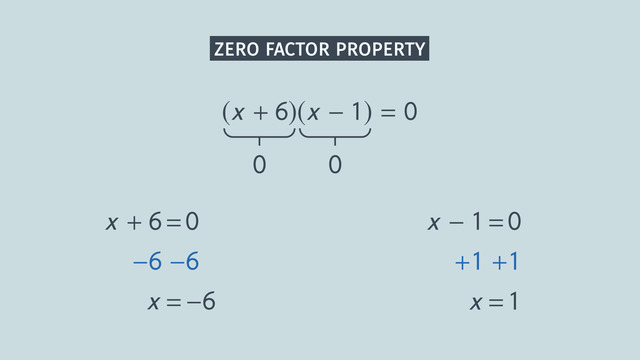
Solving Quadratic Equations by Factoring
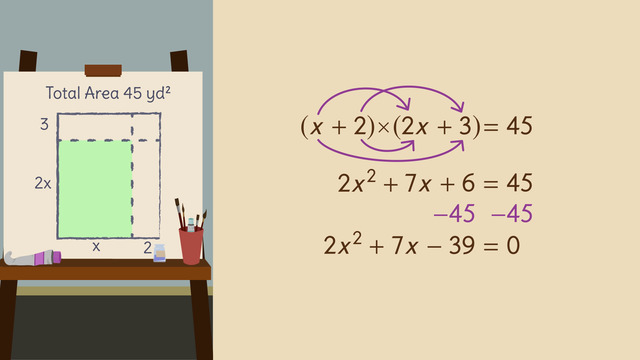
Factoring with Grouping
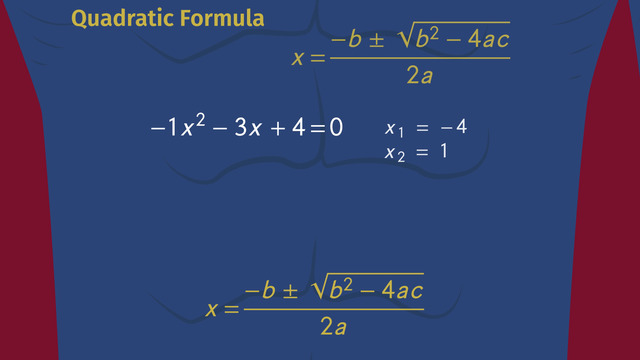
Solving Quadratic Equations Using the Quadratic Formula

Solving Quadratic Equations by Completing the Square
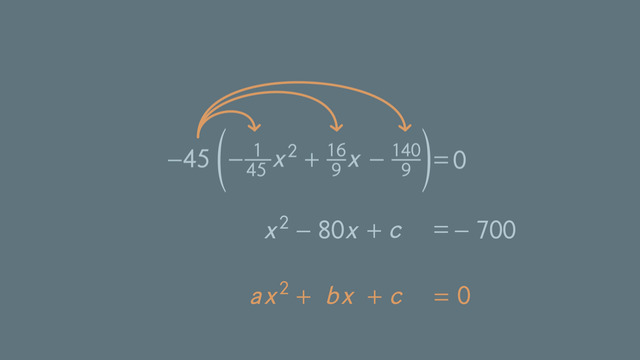
Finding the Value that Completes the Square
Using and Understanding the Discriminant

Word Problems with Quadratic Equations








